
In this section, we study Stokes’ theorem, a higher-dimensional generalization of Green’s theorem. This theorem, like the Fundamental Theorem for Line Integrals and Green’s theorem, is a generalization of the Fundamental Theorem of Calculus to higher dimensions. Stokes’ theorem relates a vector surface integral over surface S in space to a line integral around the boundary of S. Therefore, just as the theorems before it, Stokes’ theorem can be used to reduce an integral over a geometric object S to an integral over the boundary of S.
In addition to allowing us to translate between line integrals and surface integrals, Stokes’ theorem connects the concepts of curl and circulation. Furthermore, the theorem has applications in fluid mechanics and electromagnetism. We use Stokes’ theorem to derive Faraday’s law, an important result involving electric fields.
Stokes’ theorem says we can calculate the flux of curl F across surface S by knowing information only about the values of F along the boundary of S. Conversely, we can calculate the line integral of vector field F along the boundary of surface S by translating to a double integral of the curl of F over S.
Let S be an oriented smooth surface with unit normal vector N. Furthermore, suppose the boundary of S is a simple closed curve C. The orientation of S induces the positive orientation of C if, as you walk in the positive direction around C with your head pointing in the direction of N, the surface is always on your left. With this definition in place, we can state Stokes’ theorem.
Let S be a piecewise smooth oriented surface with a boundary that is a simple closed curve C with positive orientation ([link]). If F is a vector field with component functions that have continuous partial derivatives on an open region containing S, then

Suppose surface S is a flat region in the xy-plane with upward orientation. Then the unit normal vector is k and surface integral
is actually the double integral
In this special case, Stokes’ theorem gives
However, this is the flux form of Green’s theorem, which shows us that Green’s theorem is a special case of Stokes’ theorem. Green’s theorem can only handle surfaces in a plane, but Stokes’ theorem can handle surfaces in a plane or in space.
The complete proof of Stokes’ theorem is beyond the scope of this text. We look at an intuitive explanation for the truth of the theorem and then see proof of the theorem in the special case that surface S is a portion of a graph of a function, and S, the boundary of S, and F are all fairly tame.
First, we look at an informal proof of the theorem. This proof is not rigorous, but it is meant to give a general feeling for why the theorem is true. Let S be a surface and let D be a small piece of the surface so that D does not share any points with the boundary of S. We choose D to be small enough so that it can be approximated by an oriented square E. Let D inherit its orientation from S, and give E the same orientation. This square has four sides; denote them
and
for the left, right, up, and down sides, respectively. On the square, we can use the flux form of Green’s theorem:
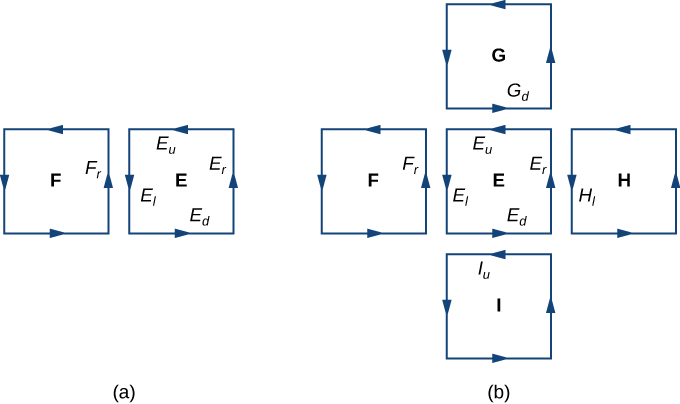
To approximate the flux over the entire surface, we add the values of the flux on the small squares approximating small pieces of the surface ([link]). By Green’s theorem, the flux across each approximating square is a line integral over its boundary. Let F be an approximating square with an orientation inherited from S and with a right side
(so F is to the left of E). Let
denote the right side of
; then,
In other words, the right side of
is the same curve as the left side of E, just oriented in the opposite direction. Therefore,
As we add up all the fluxes over all the squares approximating surface S, line integrals
and
cancel each other out. The same goes for the line integrals over the other three sides of E. These three line integrals cancel out with the line integral of the lower side of the square above E, the line integral over the left side of the square to the right of E, and the line integral over the upper side of the square below E ([link]). After all this cancelation occurs over all the approximating squares, the only line integrals that survive are the line integrals over sides approximating the boundary of S. Therefore, the sum of all the fluxes (which, by Green’s theorem, is the sum of all the line integrals around the boundaries of approximating squares) can be approximated by a line integral over the boundary of S. In the limit, as the areas of the approximating squares go to zero, this approximation gets arbitrarily close to the flux.
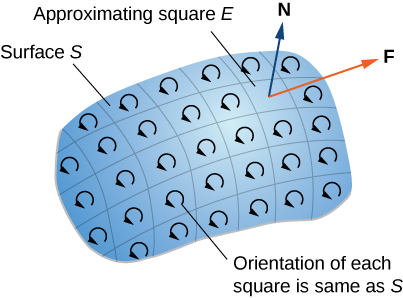
Let’s now look at a rigorous proof of the theorem in the special case that S is the graph of function
where x and y vary over a bounded, simply connected region D of finite area ([link]). Furthermore, assume that
has continuous second-order partial derivatives. Let C denote the boundary of S and let C′ denote the boundary of D. Then, D is the “shadow” of S in the plane and C′ is the “shadow” of C. Suppose that S is oriented upward. The counterclockwise orientation of C is positive, as is the counterclockwise orientation of
Let
be a vector field with component functions that have continuous partial derivatives.
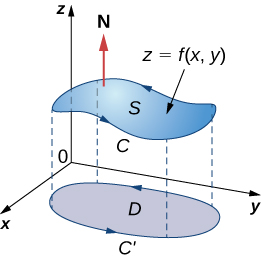
We take the standard parameterization of
The tangent vectors are
and
and therefore,
By [link],
where the partial derivatives are all evaluated at
making the integrand depend on x and y only. Suppose
is a parameterization of
Then, a parameterization of C is
Armed with these parameterizations, the Chain rule, and Green’s theorem, and keeping in mind that P, Q, and R are all functions of x and y, we can evaluate line integral
By Clairaut’s theorem,
Therefore, four of the terms disappear from this double integral, and we are left with
which equals
□
We have shown that Stokes’ theorem is true in the case of a function with a domain that is a simply connected region of finite area. We can quickly confirm this theorem for another important case: when vector field F is conservative. If F is conservative, the curl of F is zero, so
Since the boundary of S is a closed curve,
is also zero.
Verify that Stokes’ theorem is true for vector field
and surface S, where S is the paravbolid
.

Verify that Stokes’ theorem is true for vector field
and surface S, where S is the upwardly oriented portion of the graph of
over a triangle in the xy-plane with vertices
and
Both integrals give
Calculate the double integral and line integral separately.
Stokes’ theorem translates between the flux integral of surface S to a line integral around the boundary of S. Therefore, the theorem allows us to compute surface integrals or line integrals that would ordinarily be quite difficult by translating the line integral into a surface integral or vice versa. We now study some examples of each kind of translation.
Calculate surface integral
where S is the surface, oriented outward, in [link] and
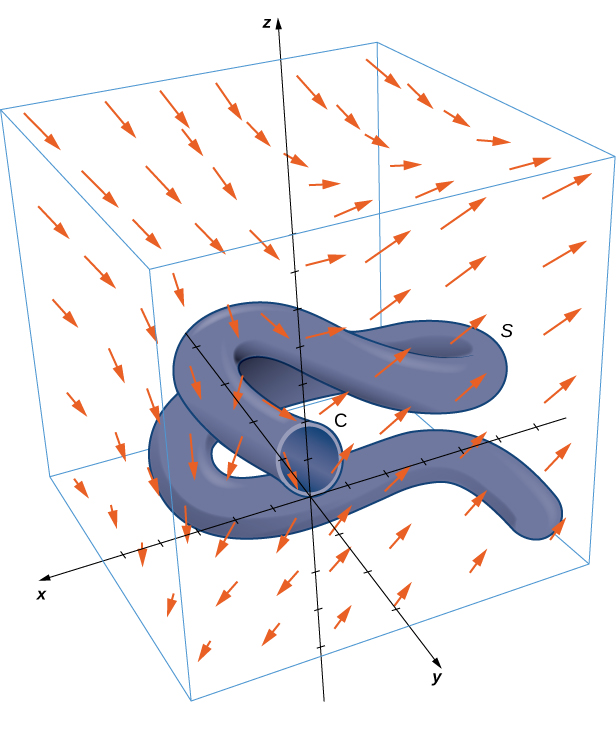
Note that to calculate
without using Stokes’ theorem, we would need to use [link]. Use of this equation requires a parameterization of S. Surface S is complicated enough that it would be extremely difficult to find a parameterization. Therefore, the methods we have learned in previous sections are not useful for this problem. Instead, we use Stokes’ theorem, noting that the boundary C of the surface is merely a single circle with radius 1.
The curl of F is
By Stokes’ theorem,
where C has parameterization
By [link],
An amazing consequence of Stokes’ theorem is that if S′ is any other smooth surface with boundary C and the same orientation as S, then
because Stokes’ theorem says the surface integral depends on the line integral around the boundary only.
In [link], we calculated a surface integral simply by using information about the boundary of the surface. In general, let
and
be smooth surfaces with the same boundary C and the same orientation. By Stokes’ theorem,
Therefore, if
is difficult to calculate but
is easy to calculate, Stokes’ theorem allows us to calculate the easier surface integral. In [link], we could have calculated
by calculating
where
is the disk enclosed by boundary curve C (a much more simple surface with which to work).
[link] shows that flux integrals of curl vector fields are surface independent in the same way that line integrals of gradient fields are path independent. Recall that if F is a two-dimensional conservative vector field defined on a simply connected domain,
is a potential function for F, and C is a curve in the domain of F, then
depends only on the endpoints of C. Therefore if C′ is any other curve with the same starting point and endpoint as C (that is, C′ has the same orientation as C), then
In other words, the value of the integral depends on the boundary of the path only; it does not really depend on the path itself.
Analogously, suppose that S and S′ are surfaces with the same boundary and same orientation, and suppose that G is a three-dimensional vector field that can be written as the curl of another vector field F (so that F is like a “potential field” of G). By [link],
Therefore, the flux integral of G does not depend on the surface, only on the boundary of the surface. Flux integrals of vector fields that can be written as the curl of a vector field are surface independent in the same way that line integrals of vector fields that can be written as the gradient of a scalar function are path independent.
Use Stokes’ theorem to calculate surface integral
where
and S is the surface as shown in the following figure. The boundary curve, C, is oriented clockwise.
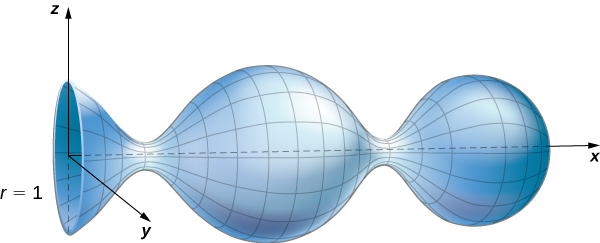
Parameterize the boundary of S and translate to a line integral.
Calculate the line integral
where
and C is the boundary of the parallelogram with vertices
and
To calculate the line integral directly, we need to parameterize each side of the parallelogram separately, calculate four separate line integrals, and add the result. This is not overly complicated, but it is time-consuming.
By contrast, let’s calculate the line integral using Stokes’ theorem. Let S denote the surface of the parallelogram. Note that S is the portion of the graph of
for
varying over the rectangular region with vertices
and
in the xy-plane. Therefore, a parameterization of S is
The curl of F is
and Stokes’ theorem and [link] give
Use Stokes’ theorem to calculate line integral
where
and C is oriented clockwise and is the boundary of a triangle with vertices
and
This triangle lies in plane
In addition to translating between line integrals and flux integrals, Stokes’ theorem can be used to justify the physical interpretation of curl that we have learned. Here we investigate the relationship between curl and circulation, and we use Stokes’ theorem to state Faraday’s law—an important law in electricity and magnetism that relates the curl of an electric field to the rate of change of a magnetic field.
Recall that if C is a closed curve and F is a vector field defined on C, then the circulation of F around C is line integral
If F represents the velocity field of a fluid in space, then the circulation measures the tendency of the fluid to move in the direction of C.
Let F be a continuous vector field and let
be a small disk of radius r with center
([link]). If
is small enough, then
for all points P in
because the curl is continuous. Let
be the boundary circle of
By Stokes’ theorem,
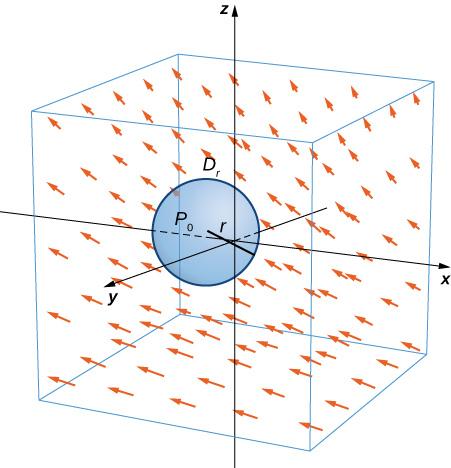
The quantity
is constant, and therefore
Thus
and the approximation gets arbitrarily close as the radius shrinks to zero. Therefore Stokes’ theorem implies that
This equation relates the curl of a vector field to the circulation. Since the area of the disk is
this equation says we can view the curl (in the limit) as the circulation per unit area. Recall that if F is the velocity field of a fluid, then circulation
is a measure of the tendency of the fluid to move around
The reason for this is that
is a component of F in the direction of T, and the closer the direction of F is to T, the larger the value of
(remember that if a and b are vectors and b is fixed, then the dot product
is maximal when a points in the same direction as b). Therefore, if F is the velocity field of a fluid, then
is a measure of how the fluid rotates about axis N. The effect of the curl is largest about the axis that points in the direction of N, because in this case
is as large as possible.
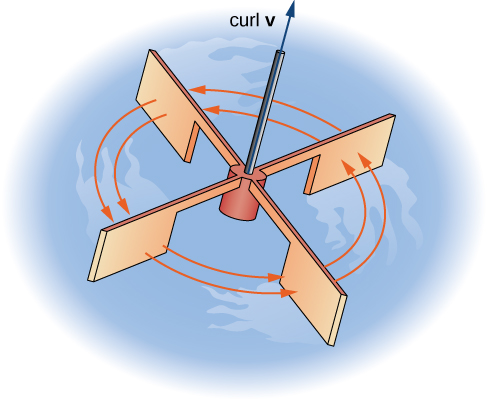
To see this effect in a more concrete fashion, imagine placing a tiny paddlewheel at point
([link]). The paddlewheel achieves its maximum speed when the axis of the wheel points in the direction of curlF. This justifies the interpretation of the curl we have learned: curl is a measure of the rotation in the vector field about the axis that points in the direction of the normal vector N, and Stokes’ theorem justifies this interpretation.
Now that we have learned about Stokes’ theorem, we can discuss applications in the area of electromagnetism. In particular, we examine how we can use Stokes’ theorem to translate between two equivalent forms of Faraday’s law. Before stating the two forms of Faraday’s law, we need some background terminology.
Let C be a closed curve that models a thin wire. In the context of electric fields, the wire may be moving over time, so we write
to represent the wire. At a given time t, curve
may be different from original curve C because of the movement of the wire, but we assume that
is a closed curve for all times t. Let
be a surface with
as its boundary, and orient
so that
has positive orientation. Suppose that
is in a magnetic field
that can also change over time. In other words, B has the form
where P, Q, and R can all vary continuously over time. We can produce current along the wire by changing field
(this is a consequence of Ampere’s law). Flux
creates electric field
that does work. The integral form of Faraday’s law states that
In other words, the work done by E is the line integral around the boundary, which is also equal to the rate of change of the flux with respect to time. The differential form of Faraday’s law states that
Using Stokes’ theorem, we can show that the differential form of Faraday’s law is a consequence of the integral form. By Stokes’ theorem, we can convert the line integral in the integral form into surface integral
Since
then as long as the integration of the surface does not vary with time we also have
Therefore,
To derive the differential form of Faraday’s law, we would like to conclude that
In general, the equation
is not enough to conclude that
The integral symbols do not simply “cancel out,” leaving equality of the integrands. To see why the integral symbol does not just cancel out in general, consider the two single-variable integrals
and
where
Both of these integrals equal
so
However,
Analogously, with our equation
we cannot simply conclude that
just because their integrals are equal. However, in our context, equation
is true for any region, however small (this is in contrast to the single-variable integrals just discussed). If F and G are three-dimensional vector fields such that
for any surface S, then it is possible to show that
by shrinking the area of S to zero by taking a limit (the smaller the area of S, the closer the value of
to the value of F at a point inside S). Therefore, we can let area
shrink to zero by taking a limit and obtain the differential form of Faraday’s law:
In the context of electric fields, the curl of the electric field can be interpreted as the negative of the rate of change of the corresponding magnetic field with respect to time.
Calculate the curl of electric field E if the corresponding magnetic field is constant field
Since the magnetic field does not change with respect to time,
By Faraday’s law, the curl of the electric field is therefore also zero.
A consequence of Faraday’s law is that the curl of the electric field corresponding to a constant magnetic field is always zero.
Calculate the curl of electric field E if the corresponding magnetic field is
Use the differential form of Faraday’s law.
Notice that the curl of the electric field does not change over time, although the magnetic field does change over time.
For the following exercises, without using Stokes’ theorem, calculate directly both the flux of
over the given surface and the circulation integral around its boundary, assuming all boundaries are oriented clockwise as viewed from above.
S is the first-octant portion of plane
S is hemisphere
S is hemisphere
S is upper hemisphere
S is a triangular region with vertices (3, 0, 0), (0, 3/2, 0), and (0, 0, 3).
S is a portion of paraboloid
and is above the xy-plane.
For the following exercises, use Stokes’ theorem to evaluate
for the vector fields and surface.
and S is the surface of the cube
except for the face where
and using the outward unit normal vector.
and C is the intersection of paraboloid
and plane
and using the outward normal vector.
and C is the intersection of sphere
with plane
and using the outward normal vector
Use Stokes’ theorem to evaluate
where C is the curve given by
traversed in the direction of increasing t.
[T] Use a computer algebraic system (CAS) and Stokes’ theorem to approximate line integral
where C is the intersection of plane
and surface
traversed counterclockwise viewed from the origin.
[T] Use a CAS and Stokes’ theorem to approximate line integral
where C is the intersection of the xy-plane and hemisphere
traversed counterclockwise viewed from the top—that is, from the positive z-axis toward the xy-plane.
[T] Use a CAS and Stokes’ theorem to approximate line integral
where C is a triangle with vertices
and
oriented counterclockwise.
Use Stokes’ theorem to evaluate
where
and S is half of sphere
oriented out toward the positive x-axis.
[T] Use a CAS and Stokes’ theorem to evaluate
where
and C is the curve of the intersection of plane
and cylinder
oriented clockwise when viewed from above.
[T] Use a CAS and Stokes’ theorem to evaluate
where
and S consists of the top and the four sides but not the bottom of the cube with vertices
oriented outward.
[T] Use a CAS and Stokes’ theorem to evaluate
where
and S is the top part of
above plane
and S is oriented upward.
Use Stokes’ theorem to evaluate
where
and S is a triangle with vertices (1, 0, 0), (0, 1, 0) and (0, 0, 1) with counterclockwise orientation.
Use Stokes’ theorem to evaluate line integral
where C is a triangle with vertices (3, 0, 0), (0, 0, 2), and (0, 6, 0) traversed in the given order.
Use Stokes’ theorem to evaluate
where C is the curve of intersection of plane
and ellipsoid
oriented clockwise from the origin.

Use Stokes’ theorem to evaluate
where
and S is the part of surface
with
oriented counterclockwise.
Use Stokes’ theorem for vector field
where S is surface
C is boundary circle
and S is oriented in the positive z-direction.
Use Stokes’ theorem for vector field
where S is that part of the surface of plane
contained within triangle C with vertices (1, 0, 0), (0, 1, 0), and (0, 0, 1), traversed counterclockwise as viewed from above.
A certain closed path C in plane
is known to project onto unit circle
in the xy-plane. Let c be a constant and let
Use Stokes’ theorem to evaluate
Use Stokes’ theorem and let C be the boundary of surface
with
and
oriented with upward facing normal. Define
Let S be hemisphere
with
oriented upward. Let
be a vector field. Use Stokes’ theorem to evaluate
Let
and let S be the graph of function
with
oriented so that the normal vector S has a positive y component. Use Stokes’ theorem to compute integral
Use Stokes’ theorem to evaluate
where
and C is a triangle with vertices (0, 0, 0), (2, 0, 0) and
oriented counterclockwise when viewed from above.
Use the surface integral in Stokes’ theorem to calculate the circulation of field F,
around C, which is the intersection of cylinder
and hemisphere
oriented counterclockwise when viewed from above.
Use Stokes’ theorem to compute
where
and S is a part of plane
inside cylinder
and oriented counterclockwise.
Use Stokes’ theorem to evaluate
where
and S is the part of plane
in the positive octant and oriented counterclockwise
Let
and let C be the intersection of plane
and cylinder
which is oriented counterclockwise when viewed from the top. Compute the line integral of F over C using Stokes’ theorem.
[T] Use a CAS and let
Use Stokes’ theorem to compute the surface integral of curl F over surface S with inward orientation consisting of cube
with the right side missing.
Let S be ellipsoid
oriented counterclockwise and let F be a vector field with component functions that have continuous partial derivatives.
Let S be the part of paraboloid
with
Verify Stokes’ theorem for vector field
[T] Use a CAS and Stokes’ theorem to evaluate
if
where C is the curve given by
[T] Use a CAS and Stokes’ theorem to evaluate
with S as a portion of paraboloid
cut off by the xy-plane oriented counterclockwise.
[T] Use a CAS to evaluate
where
and S is the surface parametrically by
Let S be paraboloid
for
where
is a real number. Let
For what value(s) of a (if any) does
have its maximum value?
For the following application exercises, the goal is to evaluate
where
and S is the upper half of ellipsoid
Evaluate a surface integral over a more convenient surface to find the value of A.
Evaluate A using a line integral.
Take paraboloid
for
and slice it with plane
Let S be the surface that remains for
including the planar surface in the xz-plane. Let C be the semicircle and line segment that bounded the cap of S in plane
with counterclockwise orientation. Let
Evaluate
For the following exercises, let S be the disk enclosed by curve
for
where
is a fixed angle.
What is the length of C in terms of
What is the circulation of C of vector field
as a function of
For what value of
is the circulation a maximum?
Circle C in plane
has radius 4 and center (2, 3, 3). Evaluate
for
where C has a counterclockwise orientation when viewed from above.
Velocity field
for
represents a horizontal flow in the y-direction. Compute the curl of v in a clockwise rotation.
Evaluate integral
where
and S is the cap of paraboloid
above plane
and n points in the positive z-direction on S.
For the following exercises, use Stokes’ theorem to find the circulation of the following vector fields around any smooth, simple closed curve C.
0

You can also download for free at http://cnx.org/contents/9a1df55a-b167-4736-b5ad-15d996704270@5.1
Attribution: