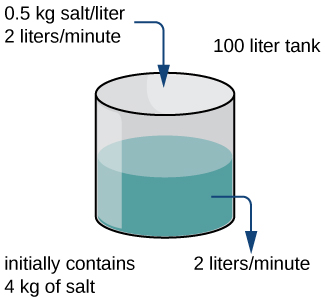
Determining Salt Concentration over Time
A tank containing
of a brine solution initially has
of salt dissolved in the solution. At time
another brine solution flows into the tank at a rate of
This brine solution contains a concentration of
of salt. At the same time, a stopcock is opened at the bottom of the tank, allowing the combined solution to flow out at a rate of
so that the level of liquid in the tank remains constant ([link]). Find the amount of salt in the tank as a function of time (measured in minutes), and find the limiting amount of salt in the tank, assuming that the solution in the tank is well mixed at all times.
First we define a function
that represents the amount of salt in kilograms in the tank as a function of time. Then
represents the rate at which the amount of salt in the tank changes as a function of time. Also,
represents the amount of salt in the tank at time
which is
kilograms.
The general setup for the differential equation we will solve is of the form
INFLOW RATE represents the rate at which salt enters the tank, and OUTFLOW RATE represents the rate at which salt leaves the tank. Because solution enters the tank at a rate of
L/min, and each liter of solution contains
kilogram of salt, every minute
of salt enters the tank. Therefore INFLOW RATE =
To calculate the rate at which salt leaves the tank, we need the concentration of salt in the tank at any point in time. Since the actual amount of salt varies over time, so does the concentration of salt. However, the volume of the solution remains fixed at 100 liters. The number of kilograms of salt in the tank at time
is equal to
Thus, the concentration of salt is
kg/L, and the solution leaves the tank at a rate of
L/min. Therefore salt leaves the tank at a rate of
kg/min, and OUTFLOW RATE is equal to
Therefore the differential equation becomes
and the initial condition is
The initial-value problem to be solved is
The differential equation is a separable equation, so we can apply the five-step strategy for solution.
Step 1. Setting
gives
as a constant solution. Since the initial amount of salt in the tank is
kilograms, this solution does not apply.
Step 2. Rewrite the equation as
Then multiply both sides by
and divide both sides by
Step 3. Integrate both sides:
Step 4. Solve for
Eliminate the absolute value by allowing the constant to be either positive or negative:
Finally, solve for
Step 5. Solve for
The solution to the initial value problem is
To find the limiting amount of salt in the tank, take the limit as
approaches infinity:
Note that this was the constant solution to the differential equation. If the initial amount of salt in the tank is
kilograms, then it remains constant. If it starts at less than 50 kilograms, then it approaches 50 kilograms over time.
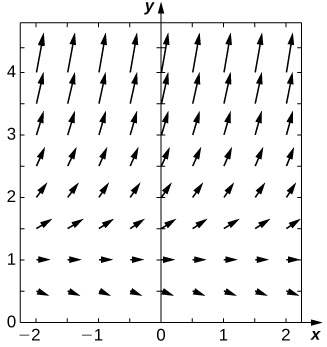
![Graph of the solution to the initial-value problem y′=(2x+3)(y2−4),y(0)=−3. A graph of the solution over [-5, 3] for x and [-3, 2] for y. It begins as a horizontal line at y = -2 from x = -5 to just before -3, almost immediately steps up to y = 2 from just after x = -3 to just before x = 0, and almost immediately steps back down to y = -2 just after x = 0 to x = 3.](../resources/CNX_Calc_Figure_08_03_001.jpg)