Is the area between the graph of
and the x-axis over the interval
finite or infinite? If this same region is revolved about the x-axis, is the volume finite or infinite? Surprisingly, the area of the region described is infinite, but the volume of the solid obtained by revolving this region about the x-axis is finite.
In this section, we define integrals over an infinite interval as well as integrals of functions containing a discontinuity on the interval. Integrals of these types are called improper integrals. We examine several techniques for evaluating improper integrals, all of which involve taking limits.
How should we go about defining an integral of the type
We can integrate
for any value of
so it is reasonable to look at the behavior of this integral as we substitute larger values of
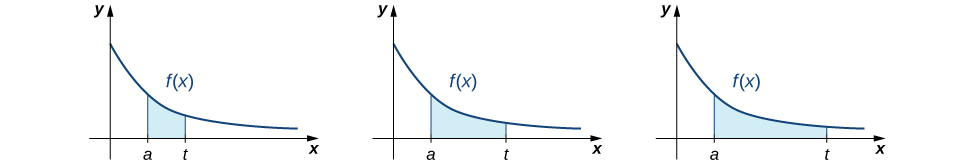
[link] shows that
may be interpreted as area for various values of
In other words, we may define an improper integral as a limit, taken as one of the limits of integration increases or decreases without bound.

be continuous over an interval of the form
Then
provided this limit exists.
be continuous over an interval of the form
Then
provided this limit exists.
In each case, if the limit exists, then the improper integral is said to converge. If the limit does not exist, then the improper integral is said to diverge.
be continuous over
Then
provided that
and
both converge. If either of these two integrals diverge, then
diverges. (It can be shown that, in fact,
for any value of
In our first example, we return to the question we posed at the start of this section: Is the area between the graph of
and the
-axis over the interval
finite or infinite?
Determine whether the area between the graph of
and the x-axis over the interval
is finite or infinite.
We first do a quick sketch of the region in question, as shown in the following graph.
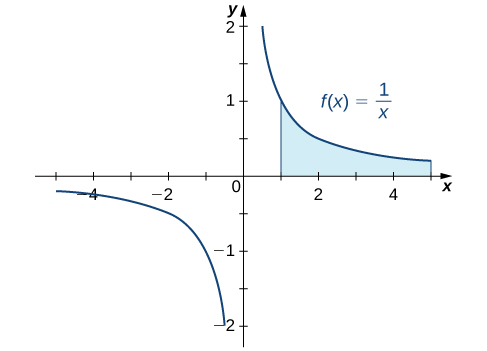
We can see that the area of this region is given by
Then we have
Since the improper integral diverges to
the area of the region is infinite.
Find the volume of the solid obtained by revolving the region bounded by the graph of
and the x-axis over the interval
about the
-axis.
The solid is shown in [link]. Using the disk method, we see that the volume V is
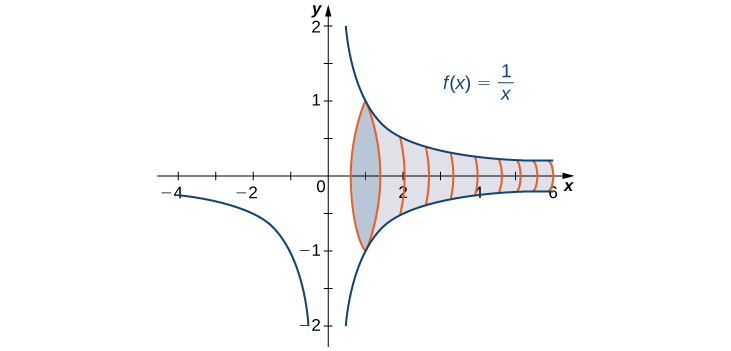
Then we have
The improper integral converges to
Therefore, the volume of the solid of revolution is
In conclusion, although the area of the region between the x-axis and the graph of
over the interval
is infinite, the volume of the solid generated by revolving this region about the x-axis is finite. The solid generated is known as Gabriel’s Horn.
Visit this website to read more about Gabriel’s Horn.

In the chapter opener, we stated the following problem: Suppose that at a busy intersection, traffic accidents occur at an average rate of one every three months. After residents complained, changes were made to the traffic lights at the intersection. It has now been eight months since the changes were made and there have been no accidents. Were the changes effective or is the 8-month interval without an accident a result of chance?
Probability theory tells us that if the average time between events is
the probability that
the time between events, is between
and
is given by
Thus, if accidents are occurring at a rate of one every 3 months, then the probability that
the time between accidents, is between
and
is given by
To answer the question, we must compute
and decide whether it is likely that 8 months could have passed without an accident if there had been no improvement in the traffic situation.
We need to calculate the probability as an improper integral:
The value
represents the probability of no accidents in 8 months under the initial conditions. Since this value is very, very small, it is reasonable to conclude the changes were effective.
Evaluate
State whether the improper integral converges or diverges.
Begin by rewriting
as a limit using [link] from the definition. Thus,
The improper integral converges to
Evaluate
State whether the improper integral converges or diverges.
Start by splitting up the integral:
If either
or
diverges, then
diverges. Compute each integral separately. For the first integral,
The first improper integral converges. For the second integral,
Thus,
diverges. Since this integral diverges,
diverges as well.
Evaluate
State whether the improper integral converges or diverges.
converges
Now let’s examine integrals of functions containing an infinite discontinuity in the interval over which the integration occurs. Consider an integral of the form
where
is continuous over
and discontinuous at
Since the function
is continuous over
for all values of
satisfying
the integral
is defined for all such values of
Thus, it makes sense to consider the values of
as
approaches
for
That is, we define
provided this limit exists. [link] illustrates
as areas of regions for values of
approaching
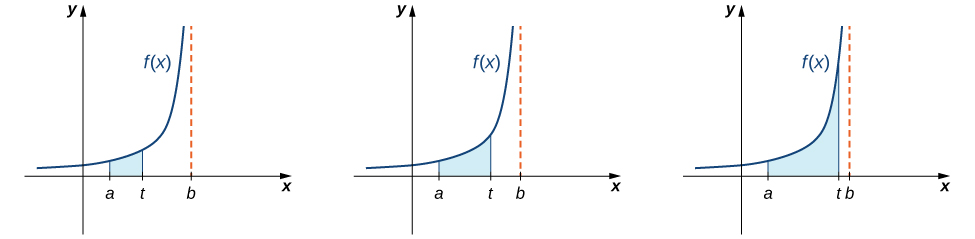
We use a similar approach to define
where
is continuous over
and discontinuous at
We now proceed with a formal definition.
be continuous over
Then,
be continuous over
Then,
In each case, if the limit exists, then the improper integral is said to converge. If the limit does not exist, then the improper integral is said to diverge.
is continuous over
except at a point
in
then
provided both
and
converge. If either of these integrals diverges, then
diverges.
The following examples demonstrate the application of this definition.
Evaluate
if possible. State whether the integral converges or diverges.
The function
is continuous over
and discontinuous at 4. Using [link] from the definition, rewrite
as a limit:
The improper integral converges.
Evaluate
State whether the integral converges or diverges.
Since
is continuous over
and is discontinuous at zero, we can rewrite the integral in limit form using [link]:
The improper integral converges.
Evaluate
State whether the improper integral converges or diverges.
Since
is discontinuous at zero, using [link], we can write
If either of the two integrals diverges, then the original integral diverges. Begin with
Therefore,
diverges. Since
diverges,
diverges.
Evaluate
State whether the integral converges or diverges.
diverges
It is not always easy or even possible to evaluate an improper integral directly; however, by comparing it with another carefully chosen integral, it may be possible to determine its convergence or divergence. To see this, consider two continuous functions
and
satisfying
for
([link]). In this case, we may view integrals of these functions over intervals of the form
as areas, so we have the relationship
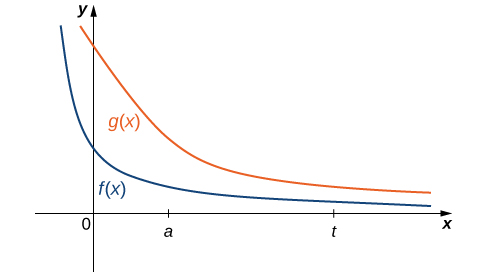
Thus, if
then
as well. That is, if the area of the region between the graph of
and the x-axis over
is infinite, then the area of the region between the graph of
and the x-axis over
is infinite too.
On the other hand, if
for some real number
then
must converge to some value less than or equal to
since
increases as
increases and
for all
If the area of the region between the graph of
and the x-axis over
is finite, then the area of the region between the graph of
and the x-axis over
is also finite.
These conclusions are summarized in the following theorem.
Let
and
be continuous over
Assume that
for
then
where
is a real number, then
for some real number
Use a comparison to show that
converges.
We can see that
so if
converges, then so does
To evaluate
first rewrite it as a limit:
Since
converges, so does
Use the comparison theorem to show that
diverges for all
Use a comparison to show that
diverges.
Since
diverges.
on
In the last few chapters, we have looked at several ways to use integration for solving real-world problems. For this next project, we are going to explore a more advanced application of integration: integral transforms. Specifically, we describe the Laplace transform and some of its properties. The Laplace transform is used in engineering and physics to simplify the computations needed to solve some problems. It takes functions expressed in terms of time and transforms them to functions expressed in terms of frequency. It turns out that, in many cases, the computations needed to solve problems in the frequency domain are much simpler than those required in the time domain.
The Laplace transform is defined in terms of an integral as
Note that the input to a Laplace transform is a function of time,
and the output is a function of frequency,
Although many real-world examples require the use of complex numbers (involving the imaginary number
in this project we limit ourselves to functions of real numbers.
Let’s start with a simple example. Here we calculate the Laplace transform of
. We have
This is an improper integral, so we express it in terms of a limit, which gives
Now we use integration by parts to evaluate the integral. Note that we are integrating with respect to t, so we treat the variable s as a constant. We have
Then we obtain
(Note, you will have to integrate by parts twice.)
Laplace transforms are often used to solve differential equations. Differential equations are not covered in detail until later in this book; but, for now, let’s look at the relationship between the Laplace transform of a function and the Laplace transform of its derivative.
Let’s start with the definition of the Laplace transform. We have
(Let
and
After integrating by parts and evaluating the limit, you should see that
Then,
Thus, differentiation in the time domain simplifies to multiplication by s in the frequency domain.
The final thing we look at in this project is how the Laplace transforms of
and its antiderivative are related. Let
Then,
(Let
and
Note, by the way, that we have defined
As you might expect, you should see that
Integration in the time domain simplifies to division by s in the frequency domain.
Evaluate the following integrals. If the integral is not convergent, answer “divergent.”
divergent
Without integrating, determine whether the integral
converges or diverges by comparing the function
with
Converges
Without integrating, determine whether the integral
converges or diverges.
Determine whether the improper integrals converge or diverge. If possible, determine the value of the integrals that converge.
Converges to 1/2.
−4
diverges
diverges
1.5
diverges
diverges
diverges
Determine the convergence of each of the following integrals by comparison with the given integral. If the integral converges, find the number to which it converges.
compare with
compare with
Both integrals diverge.
Evaluate the integrals. If the integral diverges, answer “diverges.”
diverges
diverges
0.0
0.0
Evaluate the improper integrals. Each of these integrals has an infinite discontinuity either at an endpoint or at an interior point of the interval.
6.0
Evaluate
(Be careful!) (Express your answer using three decimal places.)
Evaluate
(Express the answer in exact form.)
Evaluate
Find the area of the region in the first quadrant between the curve
and the x-axis.
Find the area of the region bounded by the curve
the x-axis, and on the left by
7.0
Find the area under the curve
bounded on the left by
Find the area under
in the first quadrant.
Find the volume of the solid generated by revolving about the x-axis the region under the curve
from
to
Find the volume of the solid generated by revolving about the y-axis the region under the curve
in the first quadrant.
Find the volume of the solid generated by revolving about the x-axis the area under the curve
in the first quadrant.
The Laplace transform of a continuous function over the interval
is defined by
(see the Student Project). This definition is used to solve some important initial-value problems in differential equations, as discussed later. The domain of F is the set of all real numbers s such that the improper integral converges. Find the Laplace transform F of each of the following functions and give the domain of F.
Use the formula for arc length to show that the circumference of the circle
is
Answers will vary.
A function is a probability density function if it satisfies the following definition:
The probability that a random variable x lies between a and b is given by
Show that
is a probability density function.
Find the probability that x is between 0 and 0.3. (Use the function defined in the preceding problem.) Use four-place decimal accuracy.
0.8775
For the following exercises, determine whether the statement is true or false. Justify your answer with a proof or a counterexample.
cannot be integrated by parts.
cannot be integrated using partial fractions.
False
In numerical integration, increasing the number of points decreases the error.
Integration by parts can always yield the integral.
False
For the following exercises, evaluate the integral using the specified method.
using integration by parts
using trigonometric substitution
using integration by parts
using partial fractions
using trigonometric substitution
using a table of integrals or a CAS
For the following exercises, integrate using whatever method you choose.
For the following exercises, approximate the integrals using the midpoint rule, trapezoidal rule, and Simpson’s rule using four subintervals, rounding to three decimals.
[T]
[T]
[T]
For the following exercises, evaluate the integrals, if possible.
for what values of
does this integral converge or diverge?
approximately 0.2194
For the following exercises, consider the gamma function given by
Show that
Extend to show that
assuming
is a positive integer.
The fastest car in the world, the Bugati Veyron, can reach a top speed of 408 km/h. The graph represents its velocity.
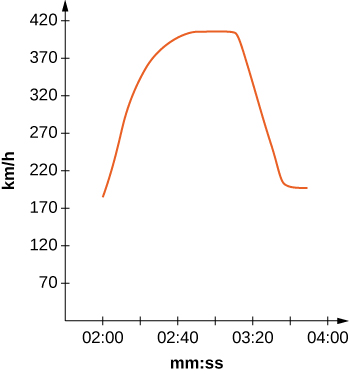
[T] Use the graph to estimate the velocity every 20 sec and fit to a graph of the form
(Hint: Consider the time units.)
[T] Using your function from the previous problem, find exactly how far the Bugati Veyron traveled in the 1 min 40 sec included in the graph.
Answers may vary. Ex:
km

You can also download for free at http://cnx.org/contents/9a1df55a-b167-4736-b5ad-15d996704270@5.1
Attribution: