Integrals Resulting in Inverse Trigonometric Functions

In this section we focus on integrals that result in inverse trigonometric functions. We have worked with these functions before. Recall from Functions and Graphs that trigonometric functions are not one-to-one unless the domains are restricted. When working with inverses of trigonometric functions, we always need to be careful to take these restrictions into account. Also in Derivatives, we developed formulas for derivatives of inverse trigonometric functions. The formulas developed there give rise directly to integration formulas involving inverse trigonometric functions.

Integrals that Result in Inverse Sine Functions

Let us begin this last section of the chapter with the three formulas. Along with these formulas, we use substitution to evaluate the integrals. We prove the formula for the inverse sine integral.

Proof

Integrals Resulting in Other Inverse Trigonometric Functions

There are six inverse trigonometric functions. However, only three integration formulas are noted in the rule on integration formulas resulting in inverse trigonometric functions because the remaining three are negative versions of the ones we use. The only difference is whether the integrand is positive or negative. Rather than memorizing three more formulas, if the integrand is negative, simply factor out −1 and evaluate the integral using one of the formulas already provided. To close this section, we examine one more formula: the integral resulting in the inverse tangent function.

Key Concepts

Key Equations

In the following exercises, evaluate each integral in terms of an inverse trigonometric function.

\int_{0}^{\sqrt{3} / 2} \frac{d x}{\sqrt{1 - x^{2}}}

{sin}^{−1} x {\|}_{0}^{\sqrt{3} / 2} = \frac{π}{3}

\int_{−1 / 2}^{1 / 2} \frac{d x}{\sqrt{1 - x^{2}}}

\int_{\sqrt{3}}^{1} \frac{d x}{\sqrt{1 + x^{2}}}

{tan}^{−1} x {\|}_{\sqrt{3}}^{1} = - \frac{π}{12}

\int_{1 / \sqrt{3}}^{\sqrt{3}} \frac{d x}{1 + x^{2}}

\int_{1}^{\sqrt{2}} \frac{d x}{\| x \| \sqrt{x^{2} - 1}}

{sec}^{−1} x {\|}_{1}^{\sqrt{2}} = \frac{π}{4}

\int_{1}^{2 / \sqrt{3}} \frac{d x}{\| x \| \sqrt{x^{2} - 1}}

In the following exercises, find each indefinite integral, using appropriate substitutions.

\int \frac{d x}{\sqrt{9 - x^{2}}}

{sin}^{−1} (\frac{x}{3}) + C

\int \frac{d x}{\sqrt{1 - 16 x^{2}}}

\int \frac{d x}{9 + x^{2}}

\frac{1}{3} {tan}^{−1} (\frac{x}{3}) + C

\int \frac{d x}{25 + 16 x^{2}}

\int \frac{d x}{\| x \| \sqrt{x^{2} - 9}}

\frac{1}{3} {sec}^{−1} (\frac{x}{3}) + C

\int \frac{d x}{\| x \| \sqrt{4 x^{2} - 16}}

Explain the relationship $− {cos}^{−1} t + C = \int \frac{d t}{\sqrt{1 - t^{2}}} = {sin}^{−1} t + C .$

Is it true, in general, that ${cos}^{−1} t = − {sin}^{−1} t ?$

cos (\frac{π}{2} - θ) = sin θ .

So, ${sin}^{−1} t = \frac{π}{2} - {cos}^{−1} t .$

They differ by a constant.

Explain the relationship ${sec}^{−1} t + C = \int \frac{d t}{\| t \| \sqrt{t^{2} - 1}} = − {csc}^{−1} t + C .$

Is it true, in general, that ${sec}^{−1} t = − {csc}^{−1} t ?$

Explain what is wrong with the following integral: $\int_{1}^{2} \frac{d t}{\sqrt{1 - t^{2}}} .$

\sqrt{1 - t^{2}}

is not defined as a real number when $t > 1 .$

Explain what is wrong with the following integral: $\int_{−1}^{1} \frac{d t}{\| t \| \sqrt{t^{2} - 1}} .$

In the following exercises, solve for the antiderivative $\int f$

of f with $C = 0,$

then use a calculator to graph f and the antiderivative over the given interval $[a, b] .$

Identify a value of C such that adding C to the antiderivative recovers the definite integral $F (x) = \int_{a}^{x} f (t) d t .$

[T] $\int \frac{1}{\sqrt{9 - x^{2}}} d x$

over $[−3, 3]$

Two graphs. The first shows the function f(x) = 1 / sqrt(9 – x^2). It is an upward opening curve symmetric about the y axis, crossing at (0, 1/3). The second shows the function F(x) = arcsin(1/3 x). It is an increasing curve going through the origin.

The antiderivative is ${sin}^{−1} (\frac{x}{3}) + C .$

Taking $C = \frac{π}{2}$

recovers the definite integral.

[T] $\int \frac{9}{9 + x^{2}} d x$

over $[−6, 6]$

[T] $\int \frac{cos x}{4 + {sin}^{2} x} d x$

over $[−6, 6]$

The antiderivative is $\frac{1}{2} {tan}^{−1} (\frac{sin x}{2}) + C .$

Taking $C = \frac{1}{2} {tan}^{−1} (\frac{sin (6)}{2})$

recovers the definite integral.

[T] $\int \frac{e^{x}}{1 + e^{2 x}} d x$

over $[−6, 6]$

In the following exercises, compute the antiderivative using appropriate substitutions.

\int \frac{{sin}^{−1} t d t}{\sqrt{1 - t^{2}}}

\frac{1}{2} {({sin}^{−1} t)}^{2} + C

\int \frac{d t}{{sin}^{−1} t \sqrt{1 - t^{2}}}

\int \frac{{tan}^{−1} (2 t)}{1 + 4 t^{2}} d t

\frac{1}{4} {({tan}^{−1} (2 t))}^{2}

\int \frac{t {tan}^{−1} (t^{2})}{1 + t^{4}} d t

\int \frac{{sec}^{−1} (\frac{t}{2})}{\| t \| \sqrt{t^{2} - 4}} d t

\frac{1}{4} ({sec}^{−1} {(\frac{t}{2})}^{2}) + C

\int \frac{t {sec}^{−1} (t^{2})}{t^{2} \sqrt{t^{4} - 1}} d t

In the following exercises, use a calculator to graph the antiderivative $\int f$

with $C = 0$

over the given interval $[a, b] .$

Approximate a value of C, if possible, such that adding C to the antiderivative gives the same value as the definite integral $F (x) = \int_{a}^{x} f (t) d t .$

[T] $\int \frac{1}{x \sqrt{x^{2} - 4}} d x$

over $[2, 6]$

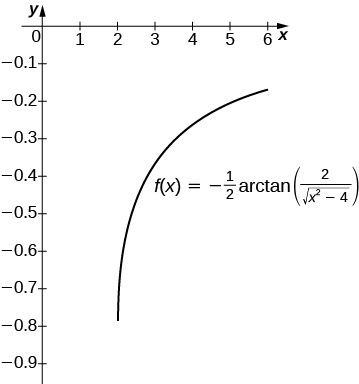
A graph of the function f(x) = -.5 * arctan(2 / ( sqrt(x^2 – 4) ) ) in quadrant four. It is an increasing concave down curve with a vertical asymptote at x=2.

The antiderivative is $\frac{1}{2} {sec}^{−1} (\frac{x}{2}) + C .$

Taking $C = 0$

recovers the definite integral over $[2, 6] .$

[T] $\int \frac{1}{(2 x + 2) \sqrt{x}} d x$

over $[0, 6]$

[T] $\int \frac{(sin x + x cos x)}{1 + x^{2} {sin}^{2} x} d x$

over $[−6, 6]$

The graph of f(x) = arctan(x sin(x)) over [-6,6]. It has five turning points at roughly (-5, -1.5), (-2,1), (0,0), (2,1), and (5,-1.5).

The general antiderivative is ${tan}^{−1} (x sin x) + C .$

Taking $C = − {tan}^{−1} (6 sin (6))$

recovers the definite integral.

[T] $\int \frac{2 e^{−2 x}}{\sqrt{1 - e^{−4 x}}} d x$

over $[0, 2]$

[T] $\int \frac{1}{x + x {ln}^{2} x}$

over $[0, 2]$

A graph of the function f(x) = arctan(ln(x)) over (0, 2]. It is an increasing curve with x-intercept at (1,0).

The general antiderivative is ${tan}^{−1} (ln x) + C .$

Taking $C = \frac{π}{2} = {tan}^{−1} \infty$

recovers the definite integral.

[T] $\int \frac{{sin}^{−1} x}{\sqrt{1 - x^{2}}}$

over $[−1, 1]$

In the following exercises, compute each integral using appropriate substitutions.

\int \frac{e^{x}}{\sqrt{1 - e^{2 t}}} d t

{sin}^{−1} (e^{t}) + C

\int \frac{e^{t}}{1 + e^{2 t}} d t

\int \frac{d t}{t \sqrt{1 - {ln}^{2} t}}

{sin}^{−1} (ln t) + C

\int \frac{d t}{t (1 + {ln}^{2} t)}

\int \frac{{cos}^{−1} (2 t)}{\sqrt{1 - 4 t^{2}}} d t

- \frac{1}{2} {({cos}^{−1} (2 t))}^{2} + C

\int \frac{e^{t} {cos}^{−1} (e^{t})}{\sqrt{1 - e^{2 t}}} d t

In the following exercises, compute each definite integral.

\int_{0}^{1 / 2} \frac{tan ({sin}^{−1} t)}{\sqrt{1 - t^{2}}} d t

\frac{1}{2} ln (\frac{4}{3})

\int_{1 / 4}^{1 / 2} \frac{tan ({cos}^{−1} t)}{\sqrt{1 - t^{2}}} d t

\int_{0}^{1 / 2} \frac{sin ({tan}^{−1} t)}{1 + t^{2}} d t

1 - \frac{2}{\sqrt{5}}

\int_{0}^{1 / 2} \frac{cos ({tan}^{−1} t)}{1 + t^{2}} d t

For $A > 0,$

compute $I (A) = \int_{− A}^{A} \frac{d t}{1 + t^{2}}$

and evaluate $lim_{a \to \infty} I (A),$

the area under the graph of $\frac{1}{1 + t^{2}}$

on $[− \infty, \infty] .$

2 {tan}^{−1} (A) \to π

as $A \to \infty$

For $1 < B < \infty,$

compute $I (B) = \int_{1}^{B} \frac{d t}{t \sqrt{t^{2} - 1}}$

and evaluate $lim_{B \to \infty} I (B),$

the area under the graph of $\frac{1}{t \sqrt{t^{2} - 1}}$

over $[1, \infty) .$

Use the substitution $u = \sqrt{2} cot x$

and the identity $1 + {cot}^{2} x = {csc}^{2} x$

to evaluate $\int \frac{d x}{1 + {cos}^{2} x} .$

(Hint: Multiply the top and bottom of the integrand by ${csc}^{2} x .)$

Using the hint, one has $\int \frac{{csc}^{2} x}{{csc}^{2} x + {cot}^{2} x} d x = \int \frac{{csc}^{2} x}{1 + 2 {cot}^{2} x} d x .$

Set $u = \sqrt{2} cot x .$

Then, $d u = − \sqrt{2} {csc}^{2} x$

and the integral is $- \frac{1}{\sqrt{2}} \int \frac{d u}{1 + u^{2}} = - \frac{1}{\sqrt{2}} {tan}^{−1} u + C = \frac{1}{\sqrt{2}} {tan}^{−1} (\sqrt{2} cot x) + C .$

If one uses the identity ${tan}^{−1} s + {tan}^{−1} (\frac{1}{s}) = \frac{π}{2},$

then this can also be written $\frac{1}{\sqrt{2}} {tan}^{−1} (\frac{tan x}{\sqrt{2}}) + C .$

[T] Approximate the points at which the graphs of $f (x) = 2 x^{2} - 1$

and $g (x) = {(1 + 4 x^{2})}^{−3 / 2}$

intersect, and approximate the area between their graphs accurate to three decimal places.

47. [T] Approximate the points at which the graphs of $f (x) = x^{2} - 1$

and $f (x) = x^{2} - 1$

intersect, and approximate the area between their graphs accurate to three decimal places.

x \approx \pm 1.13525 .

The left endpoint estimate with $N = 100$

is 2.796 and these decimals persist for $N = 500 .$

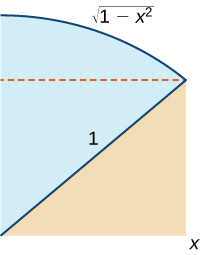
Use the following graph to prove that $\int_{0}^{x} \sqrt{1 - t^{2}} d t = \frac{1}{2} x \sqrt{1 - x^{2}} + \frac{1}{2} {sin}^{−1} x .$

Chapter Review Exercises

True or False. Justify your answer with a proof or a counterexample. Assume all functions

f

for the following functions over the specified interval. Compare your answer with the exact answer, when possible, or use a calculator to determine the answer.

The following problems consider the historic average cost per gigabyte of RAM on a computer.

| Year | 5-Year Change ($) | {: valign=”top”}|———- | 1980 | 0 | {: valign=”top”}| 1985 | −5,468,750 | {: valign=”top”}| 1990 | −755,495 | {: valign=”top”}| 1995 | −73,005 | {: valign=”top”}| 2000 | −29,768 | {: valign=”top”}| 2005 | −918 | {: valign=”top”}| 2010 | −177 | {: valign=”top”}{: .unnumbered summary=”A table with two columns and eight rows. The first column has the label “Year” and the values 1980, 1985, 1990, 1995, 2000, 2005, and 2010. The second column has the label “5-Tear Change ($)” and the values 0, -5,468,750, -755,495, -73,005, -29,768, -918, and -177.” data-label=””}