Substitution

The Fundamental Theorem of Calculus gave us a method to evaluate integrals without using Riemann sums. The drawback of this method, though, is that we must be able to find an antiderivative, and this is not always easy. In this section we examine a technique, called integration by substitution, to help us find antiderivatives. Specifically, this method helps us find antiderivatives when the integrand is the result of a chain-rule derivative.

At first, the approach to the substitution procedure may not appear very obvious. However, it is primarily a visual task—that is, the integrand shows you what to do; it is a matter of recognizing the form of the function. So, what are we supposed to see? We are looking for an integrand of the form

f [g (x)] g^{'} (x) d x .

The method is called substitution because we substitute part of the integrand with the variable u and part of the integrand with du. It is also referred to as change of variables because we are changing variables to obtain an expression that is easier to work with for applying the integration rules.

Proof

Sometimes we need to adjust the constants in our integral if they don’t match up exactly with the expressions we are substituting.

Sometimes we need to manipulate an integral in ways that are more complicated than just multiplying or dividing by a constant. We need to eliminate all the expressions within the integrand that are in terms of the original variable. When we are done, u should be the only variable in the integrand. In some cases, this means solving for the original variable in terms of u. This technique should become clear in the next example.

Substitution for Definite Integrals

Substitution can be used with definite integrals, too. However, using substitution to evaluate a definite integral requires a change to the limits of integration. If we change variables in the integrand, the limits of integration change as well.

Although we will not formally prove this theorem, we justify it with some calculations here. From the substitution rule for indefinite integrals, if

F (x)

Substitution may be only one of the techniques needed to evaluate a definite integral. All of the properties and rules of integration apply independently, and trigonometric functions may need to be rewritten using a trigonometric identity before we can apply substitution. Also, we have the option of replacing the original expression for u after we find the antiderivative, which means that we do not have to change the limits of integration. These two approaches are shown in [link].

Key Concepts

Key Equations

Why is u-substitution referred to as change of variable?

2. If $f = g \circ h,$

when reversing the chain rule, $\frac{d}{d x} (g \circ h) (x) = g^{'} (h (x)) h^{'} (x),$

should you take $u = g (x)$

or $u = h (x) ?$

u = h (x)

In the following exercises, verify each identity using differentiation. Then, using the indicated u-substitution, identify f such that the integral takes the form $\int f (u) d u .$

\int x \sqrt{x + 1} d x = \frac{2}{15} {(x + 1)}^{3 / 2} (3 x - 2) + C; u = x + 1

\int \frac{x^{2}}{\sqrt{x - 1}} d x (x > 1) = \frac{2}{15} \sqrt{x - 1} (3 x^{2} + 4 x + 8) + C; u = x - 1

f (u) = \frac{{(u + 1)}^{2}}{\sqrt{u}}

\int x \sqrt{4 x^{2} + 9} d x = \frac{1}{12} {(4 x^{2} + 9)}^{3 / 2} + C; u = 4 x^{2} + 9

\int \frac{x}{\sqrt{4 x^{2} + 9}} d x = \frac{1}{4} \sqrt{4 x^{2} + 9} + C; u = 4 x^{2} + 9

d u = 8 x d x; f (u) = \frac{1}{8 \sqrt{u}}

\int \frac{x}{{(4 x^{2} + 9)}^{2}} d x = - \frac{1}{8 (4 x^{2} + 9)}; u = 4 x^{2} + 9

In the following exercises, find the antiderivative using the indicated substitution.

\int {(x + 1)}^{4} d x; u = x + 1

\frac{1}{5} {(x + 1)}^{5} + C

\int {(x - 1)}^{5} d x; u = x - 1

\int {(2 x - 3)}^{−7} d x; u = 2 x - 3

- \frac{1}{12 {(3 - 2 x)}^{6}} + C

\int {(3 x - 2)}^{−11} d x; u = 3 x - 2

\int \frac{x}{\sqrt{x^{2} + 1}} d x; u = x^{2} + 1

\sqrt{x^{2} + 1} + C

\int \frac{x}{\sqrt{1 - x^{2}}} d x; u = 1 - x^{2}

\int (x - 1) {(x^{2} - 2 x)}^{3} d x; u = x^{2} - 2 x

\frac{1}{8} {(x^{2} - 2 x)}^{4} + C

\int (x^{2} - 2 x) {(x^{3} - 3 x^{2})}^{2} d x; u = x^{3} = 3 x^{2}

\int {cos}^{3} θ d θ; u = sin θ

(H i n t : {cos}^{2} θ = 1 - {sin}^{2} θ)

sin θ - \frac{{sin}^{3} θ}{3} + C

\int {sin}^{3} θ d θ; u = cos θ

(H i n t : {sin}^{2} θ = 1 - {cos}^{2} θ)

In the following exercises, use a suitable change of variables to determine the indefinite integral.

\int x {(1 - x)}^{99} d x

\frac{{(1 - x)}^{101}}{101} - \frac{{(1 - x)}^{100}}{100} + C

\int t {(1 - t^{2})}^{10} d t

\int {(11 x - 7)}^{−3} d x

- \frac{1}{22 (7 - 11 x^{2})} + C

\int {(7 x - 11)}^{4} d x

\int {cos}^{3} θ sin θ d θ

- \frac{{cos}^{4} θ}{4} + C

\int {sin}^{7} θ cos θ d θ

\int {cos}^{2} (π t) sin (π t) d t

- \frac{{cos}^{3} (π t)}{3 π} + C

\int {sin}^{2} x {cos}^{3} x d x

(H i n t : {sin}^{2} x + {cos}^{2} x = 1)

\int t sin (t^{2}) cos (t^{2}) d t

- \frac{1}{4} {cos}^{2} (t^{2}) + C

\int t^{2} {cos}^{2} (t^{3}) sin (t^{3}) d t

\int \frac{x^{2}}{{(x^{3} - 3)}^{2}} d x

- \frac{1}{3 (x^{3} - 3)} + C

\int \frac{x^{3}}{\sqrt{1 - x^{2}}} d x

\int \frac{y^{5}}{{(1 - y^{3})}^{3 / 2}} d y

- \frac{2 (y^{3} - 2)}{3 \sqrt{1 - y^{3}}}

{\int cos θ (1 - cos θ)}^{99} sin θ d θ

{\int (1 - {cos}^{3} θ)}^{10} {cos}^{2} θ sin θ d θ

\frac{1}{33} {(1 - {cos}^{3} θ)}^{11} + C

\int (cos θ - 1) {({cos}^{2} θ - 2 cos θ)}^{3} sin θ d θ

\int ({sin}^{2} θ - 2 sin θ) {({sin}^{3} θ - 3 {sin}^{2} θ)}^{3} cos θ d θ

\frac{1}{12} {({sin}^{3} θ - 3 {sin}^{2} θ)}^{4} + C

In the following exercises, use a calculator to estimate the area under the curve using left Riemann sums with 50 terms, then use substitution to solve for the exact answer.

[T] $y = 3 {(1 - x)}^{2}$

over $[0, 2]$

[T] $y = x {(1 - x^{2})}^{3}$

over $[−1, 2]$

L_{50} = −8.5779 .

The exact area is $\frac{−81}{8}$

[T] $y = sin x {(1 - cos x)}^{2}$

over $[0, π]$

[T] $y = \frac{x}{{(x^{2} + 1)}^{2}}$

over $[−1, 1]$

L_{50} = −0.006399

… The exact area is 0.

In the following exercises, use a change of variables to evaluate the definite integral.

\int_{0}^{1} x \sqrt{1 - x^{2}} d x

\int_{0}^{1} \frac{x}{\sqrt{1 + x^{2}}} d x

u = 1 + x^{2}, d u = 2 x d x, \frac{1}{2} \int_{1}^{2} u^{−1 / 2} d u = \sqrt{2} - 1

\int_{0}^{2} \frac{t}{\sqrt{5 + t^{2}}} d t

\int_{0}^{1} \frac{t^{2}}{\sqrt{1 + t^{3}}} d t

u = 1 + t^{3}, d u = 3 t^{2}, \frac{1}{3} \int_{1}^{2} u^{−1 / 2} d u = \frac{2}{3} (\sqrt{2} - 1)

\int_{0}^{π / 4} {sec}^{2} θ tan θ d θ

\int_{0}^{π / 4} \frac{sin θ}{{cos}^{4} θ} d θ

u = cos θ, d u = − sin θ d θ, \int_{1 / \sqrt{2}}^{1} u^{−4} d u = \frac{1}{3} (2 \sqrt{2} - 1)

In the following exercises, evaluate the indefinite integral $\int f (x) d x$

with constant $C = 0$

using u-substitution. Then, graph the function and the antiderivative over the indicated interval. If possible, estimate a value of C that would need to be added to the antiderivative to make it equal to the definite integral $F (x) = \int_{a}^{x} f (t) d t,$

with a the left endpoint of the given interval.

[T] $\int (2 x + 1) e^{x^{2} + x - 6} d x$

over $[−3, 2]$

[T] $\int \frac{cos (ln (2 x))}{x} d x$

on $[0, 2]$

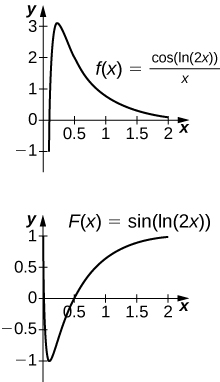
Two graphs. The first shows the function f(x) = cos(ln(2x)) / x, which increases sharply over the approximate interval (0,.25) and then decreases gradually to the x axis. The second shows the function f(x) = sin(ln(2x)), which decreases sharply on the approximate interval (0, .25) and then increases in a gently curve into the first quadrant.

The antiderivative is $y = sin (ln (2 x)) .$

Since the antiderivative is not continuous at $x = 0,$

one cannot find a value of C that would make $y = sin (ln (2 x)) - C$

work as a definite integral.

[T] $\int \frac{3 x^{2} + 2 x + 1}{\sqrt{x^{3} + x^{2} + x + 4}} d x$

over $[−1, 2]$

[T] $\int \frac{sin x}{{cos}^{3} x} d x$

over $[- \frac{π}{3}, \frac{π}{3}]$

The antiderivative is $y = \frac{1}{2} {sec}^{2} x .$

You should take $C = −2$

so that $F (- \frac{π}{3}) = 0 .$

[T] $\int (x + 2) e^{− x^{2} - 4 x + 3} d x$

over $[−5, 1]$

[T] $\int 3 x^{2} \sqrt{2 x^{3} + 1} d x$

over $[0, 1]$

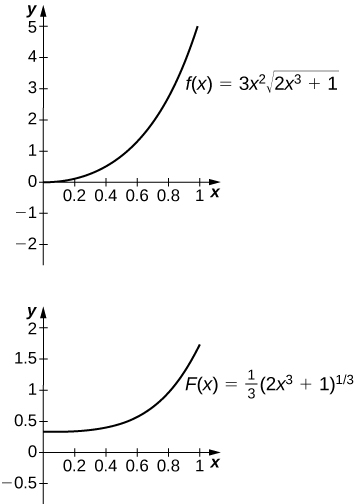
Two graphs. The first shows the function f(x) = 3x^2 * sqrt(2x^3 + 1). It is an increasing concave up curve starting at the origin. The second shows the function f(x) = 1/3 * (2x^3 + 1)^(1/3). It is an increasing concave up curve starting at about 0.3.

The antiderivative is $y = \frac{1}{3} {(2 x^{3} + 1)}^{3 / 2} .$

One should take $C = - \frac{1}{3} .$

If $h (a) = h (b)$

in $\int_{a}^{b} g' (h (x)) h (x) d x,$

what can you say about the value of the integral?

Is the substitution $u = 1 - x^{2}$

in the definite integral $\int_{0}^{2} \frac{x}{1 - x^{2}} d x$

okay? If not, why not?

No, because the integrand is discontinuous at $x = 1 .$

In the following exercises, use a change of variables to show that each definite integral is equal to zero.

\int_{0}^{π} {cos}^{2} (2 θ) sin (2 θ) d θ

\int_{0}^{\sqrt{π}} t cos (t^{2}) sin (t^{2}) d t

u = sin (t^{2});

the integral becomes $\frac{1}{2} \int_{0}^{0} u d u .$

\int_{0}^{1} (1 - 2 t) d t

\int_{0}^{1} \frac{1 - 2 t}{(1 + {(t - \frac{1}{2})}^{2})} d t

u = (1 + {(t - \frac{1}{2})}^{2});

the integral becomes $− \int_{5 / 4}^{5 / 4} \frac{1}{u} d u .$

\int_{0}^{π} sin ({(t - \frac{π}{2})}^{3}) cos (t - \frac{π}{2}) d t

\int_{0}^{2} (1 - t) cos (π t) d t

u = 1 - t;

the integral becomes* * *

\begin{array}{l} \int_{1}^{−1} u cos (π (1 - u)) d u \\ = \int_{1}^{−1} u [cos π cos u - sin π sin u] d u \\ = − \int_{1}^{−1} u cos u d u \\ = \int_{−1}^{1} u cos u d u = 0 \end{array}

since the integrand is odd.

\int_{π / 4}^{3 π / 4} {sin}^{2} t cos t d t

Show that the average value of $f (x)$

over an interval $[a, b]$

is the same as the average value of $f (c x)$

over the interval $[\frac{a}{c}, \frac{b}{c}]$

for $c > 0 .$

Setting $u = c x$

and $d u = c d x$

gets you $\frac{1}{\frac{b}{c} - \frac{a}{c}} \int_{a / c}^{b / c} f (c x) d x = \frac{c}{b - a} \int_{u = a}^{u = b} f (u) \frac{d u}{c} = \frac{1}{b - a} \int_{a}^{b} f (u) d u .$

Find the area under the graph of $f (t) = \frac{t}{{(1 + t^{2})}^{a}}$

between $t = 0$

and $t = x$

where $a > 0$

and $a \neq 1$

is fixed, and evaluate the limit as $x \to \infty .$

Find the area under the graph of $g (t) = \frac{t}{{(1 - t^{2})}^{a}}$

between $t = 0$

and $t = x,$

where $0 < x < 1$

and $a > 0$

is fixed. Evaluate the limit as $x \to 1 .$

\int_{0}^{x} g (t) d t = \frac{1}{2} \int_{u = 1 - x^{2}}^{1} \frac{d u}{u^{a}} = \frac{1}{2 (1 - a)} u^{1 - a} {\|}_{u = 1 - x^{2}}^{1} = \frac{1}{2 (1 - a)} (1 - {(1 - x^{2})}^{1 - a}) .

As $x \to 1$

the limit is $\frac{1}{2 (1 - a)}$

if $a < 1,$

and the limit diverges to +∞ if $a > 1 .$

The area of a semicircle of radius 1 can be expressed as $\int_{−1}^{1} \sqrt{1 - x^{2}} d x .$

Use the substitution $x = cos t$

to express the area of a semicircle as the integral of a trigonometric function. You do not need to compute the integral.

The area of the top half of an ellipse with a major axis that is the x-axis from $x = −1$

to a and with a minor axis that is the y-axis from $y = − b$

to b can be written as $\int_{− a}^{a} b \sqrt{1 - \frac{x^{2}}{a^{2}}} d x .$

Use the substitution $x = a cos t$

to express this area in terms of an integral of a trigonometric function. You do not need to compute the integral.

\int_{t = π}^{0} b \sqrt{1 - {cos}^{2} t} \times (− a sin t) d t = \int_{t = 0}^{π} a b {sin}^{2} t d t

[T] The following graph is of a function of the form $f (t) = a sin (n t) + b sin (m t) .$

Estimate the coefficients a and b, and the frequency parameters n and m. Use these estimates to approximate $\int_{0}^{π} f (t) d t .$

[T] The following graph is of a function of the form $f (x) = a cos (n t) + b cos (m t) .$

Estimate the coefficients a and b and the frequency parameters n and m. Use these estimates to approximate $\int_{0}^{π} f (t) d t .$

f (t) = 2 cos (3 t) - cos (2 t); \int_{0}^{π / 2} (2 cos (3 t) - cos (2 t)) = - \frac{2}{3}

Proof

Substitution for Definite Integrals

Key Concepts

Key Equations

Glossary