One of the most important types of motion in physics is simple harmonic motion, which is associated with such systems as an object with mass oscillating on a spring. Simple harmonic motion can be described by using either sine or cosine functions. In this section we expand our knowledge of derivative formulas to include derivatives of these and other trigonometric functions. We begin with the derivatives of the sine and cosine functions and then use them to obtain formulas for the derivatives of the remaining four trigonometric functions. Being able to calculate the derivatives of the sine and cosine functions will enable us to find the velocity and acceleration of simple harmonic motion.
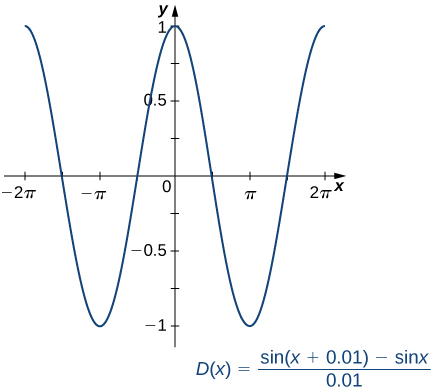
We begin our exploration of the derivative for the sine function by using the formula to make a reasonable guess at its derivative. Recall that for a function
Consequently, for values of
very close to 0,
We see that by using
By setting
and using a graphing utility, we can get a graph of an approximation to the derivative of
([link]).

Upon inspection, the graph of
appears to be very close to the graph of the cosine function. Indeed, we will show that
If we were to follow the same steps to approximate the derivative of the cosine function, we would find that
The derivative of the sine function is the cosine and the derivative of the cosine function is the negative sine.
Because the proofs for
and
use similar techniques, we provide only the proof for
Before beginning, recall two important trigonometric limits we learned in Introduction to Limits:
The graphs of
and
are shown in [link].
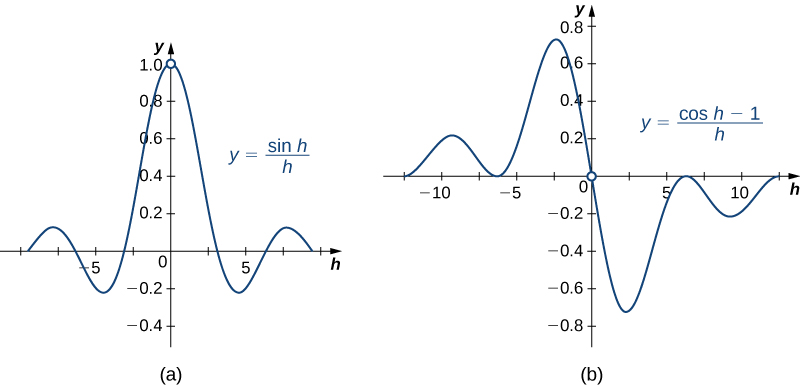
We also recall the following trigonometric identity for the sine of the sum of two angles:
Now that we have gathered all the necessary equations and identities, we proceed with the proof.
□
[link] shows the relationship between the graph of
and its derivative
Notice that at the points where
has a horizontal tangent, its derivative
takes on the value zero. We also see that where
is increasing,
and where
is decreasing,
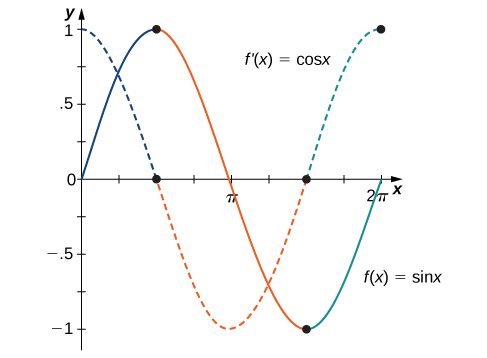
Find the derivative of
Using the product rule, we have
After simplifying, we obtain
Find the derivative of
Don’t forget to use the product rule.
Find the derivative of
By applying the quotient rule, we have
Simplifying, we obtain
Find the derivative of
Use the quotient rule.
A particle moves along a coordinate axis in such a way that its position at time
is given by
for
At what times is the particle at rest?
To determine when the particle is at rest, set
Begin by finding
We obtain
so we must solve
The solutions to this equation are
and
Thus the particle is at rest at times
and
A particle moves along a coordinate axis. Its position at time
is given by
for
At what times is the particle at rest?
Use the previous example as a guide.
Since the remaining four trigonometric functions may be expressed as quotients involving sine, cosine, or both, we can use the quotient rule to find formulas for their derivatives.
Find the derivative of
Start by expressing
as the quotient of
and
Now apply the quotient rule to obtain
Simplifying, we obtain
Recognizing that
by the Pythagorean theorem, we now have
Finally, use the identity
to obtain
Find the derivative of
Rewrite
as
and use the quotient rule.
The derivatives of the remaining trigonometric functions may be obtained by using similar techniques. We provide these formulas in the following theorem.
The derivatives of the remaining trigonometric functions are as follows:
Find the equation of a line tangent to the graph of
at
To find the equation of the tangent line, we need a point and a slope at that point. To find the point, compute
Thus the tangent line passes through the point
Next, find the slope by finding the derivative of
and evaluating it at
Using the point-slope equation of the line, we obtain
or equivalently,
Find the derivative of
To find this derivative, we must use both the sum rule and the product rule. Using the sum rule, we find
In the first term,
and by applying the product rule to the second term we obtain
Therefore, we have
Find the derivative of
Use the rule for differentiating a constant multiple and the rule for differentiating a difference of two functions.
Find the slope of the line tangent to the graph of
at
Evaluate the derivative at
The higher-order derivatives of
and
follow a repeating pattern. By following the pattern, we can find any higher-order derivative of
and
Find the first four derivatives of
Each step in the chain is straightforward:
Once we recognize the pattern of derivatives, we can find any higher-order derivative by determining the step in the pattern to which it corresponds. For example, every fourth derivative of sin x equals sin x, so
For
find
See the previous example.
Find
We can see right away that for the 74th derivative of
so
For
find
A particle moves along a coordinate axis in such a way that its position at time
is given by
Find
and
Compare these values and decide whether the particle is speeding up or slowing down.
First find
Thus,
Next, find
Thus,
and we have
Since
and
we see that velocity and acceleration are acting in opposite directions; that is, the object is being accelerated in the direction opposite to the direction in which it is travelling. Consequently, the particle is slowing down.
A block attached to a spring is moving vertically. Its position at time
is given by
Find
and
Compare these values and decide whether the block is speeding up or slowing down.
and
The block is speeding up.
Use [link] as a guide.
For the following exercises, find
for the given functions.
For the following exercises, find the equation of the tangent line to each of the given functions at the indicated values of
Then use a calculator to graph both the function and the tangent line to ensure the equation for the tangent line is correct.
[T]
[T]
[T]

[T]
[T]
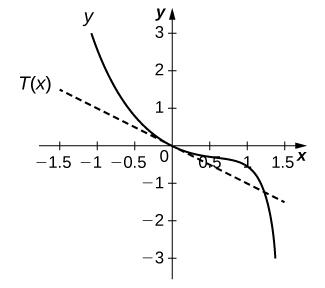
[T]
For the following exercises, find
for the given functions.
Find all
values on the graph of
where the tangent line is horizontal.
Find all
values on the graph of
for
where the tangent line has slope 2.
Let
Determine the points on the graph of
for
where the tangent line(s) is (are) parallel to the line
[T] A mass on a spring bounces up and down in simple harmonic motion, modeled by the function
where
is measured in inches and
is measured in seconds. Find the rate at which the spring is oscillating at
s.
Let the position of a swinging pendulum in simple harmonic motion be given by
Find the constants
and
such that when the velocity is 3 cm/s,
and
After a diver jumps off a diving board, the edge of the board oscillates with position given by
cm at
seconds after the jump.
The number of hamburgers sold at a fast-food restaurant in Pasadena, California, is given by
where
is the number of hamburgers sold and
represents the number of hours after the restaurant opened at 11 a.m. until 11 p.m., when the store closes. Find
and determine the intervals where the number of burgers being sold is increasing.
increasing on
and
[T] The amount of rainfall per month in Phoenix, Arizona, can be approximated by
where
is months since January. Find
and use a calculator to determine the intervals where the amount of rain falling is decreasing.
For the following exercises, use the quotient rule to derive the given equations.
Use the definition of derivative and the identity
to prove that
For the following exercises, find the requested higher-order derivative for the given functions.
of
of
of
of
of

You can also download for free at http://cnx.org/contents/9a1df55a-b167-4736-b5ad-15d996704270@5.1
Attribution: