
Many functions have the property that their graphs can be traced with a pencil without lifting the pencil from the page. Such functions are called continuous. Other functions have points at which a break in the graph occurs, but satisfy this property over intervals contained in their domains. They are continuous on these intervals and are said to have a discontinuity at a point where a break occurs.
We begin our investigation of continuity by exploring what it means for a function to have continuity at a point. Intuitively, a function is continuous at a particular point if there is no break in its graph at that point.
Before we look at a formal definition of what it means for a function to be continuous at a point, let’s consider various functions that fail to meet our intuitive notion of what it means to be continuous at a point. We then create a list of conditions that prevent such failures.
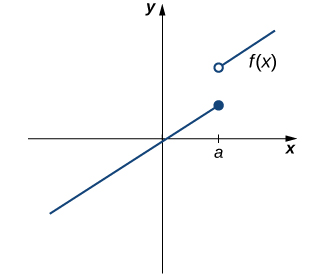
Our first function of interest is shown in [link]. We see that the graph of
has a hole at a. In fact,
is undefined. At the very least, for
to be continuous at a, we need the following condition:

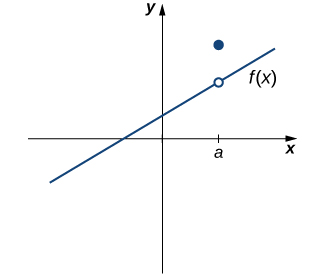
However, as we see in [link], this condition alone is insufficient to guarantee continuity at the point a. Although
is defined, the function has a gap at a. In this example, the gap exists because
does not exist. We must add another condition for continuity at a—namely,
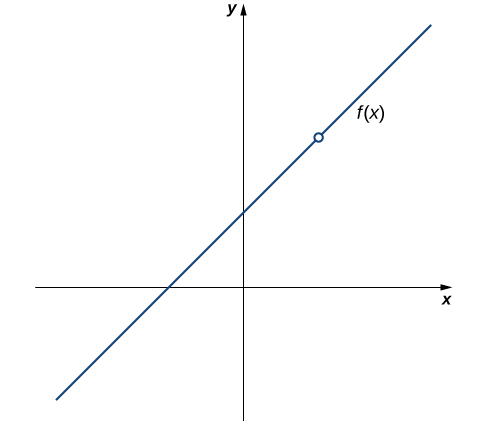
However, as we see in [link], these two conditions by themselves do not guarantee continuity at a point. The function in this figure satisfies both of our first two conditions, but is still not continuous at a. We must add a third condition to our list:
Now we put our list of conditions together and form a definition of continuity at a point.
A function
is continuous at a point a if and only if the following three conditions are satisfied:
is defined
exists
A function is discontinuous at a point a if it fails to be continuous at a.
The following procedure can be used to analyze the continuity of a function at a point using this definition.
is defined. If
is undefined, we need go no further. The function is not continuous at a. If
is defined, continue to step 2.
In some cases, we may need to do this by first computing
and
If
does not exist (that is, it is not a real number), then the function is not continuous at a and the problem is solved. If
exists, then continue to step 3.
and
If
then the function is not continuous at a. If
then the function is continuous at a.
The next three examples demonstrate how to apply this definition to determine whether a function is continuous at a given point. These examples illustrate situations in which each of the conditions for continuity in the definition succeed or fail.
Using the definition, determine whether the function
is continuous at
Justify the conclusion.
Let’s begin by trying to calculate
We can see that
which is undefined. Therefore,
is discontinuous at 2 because
is undefined. The graph of
is shown in [link].
Using the definition, determine whether the function
is continuous at
Justify the conclusion.
Let’s begin by trying to calculate
Thus,
is defined. Next, we calculate
To do this, we must compute
and
and
Therefore,
does not exist. Thus,
is not continuous at 3. The graph of
is shown in [link].
Using the definition, determine whether the function
is continuous at
First, observe that
Next,
Last, compare
and
We see that
Since all three of the conditions in the definition of continuity are satisfied,
is continuous at
Using the definition, determine whether the function
is continuous at
If the function is not continuous at 1, indicate the condition for continuity at a point that fails to hold.
f is not continuous at 1 because
Check each condition of the definition.
By applying the definition of continuity and previously established theorems concerning the evaluation of limits, we can state the following theorem.
Polynomials and rational functions are continuous at every point in their domains.
Previously, we showed that if
and
are polynomials,
for every polynomial
and
as long as
Therefore, polynomials and rational functions are continuous on their domains.
□
We now apply [link] to determine the points at which a given rational function is continuous.
For what values of x is
continuous?
The rational function
is continuous for every value of x except
As we have seen in [link] and [link], discontinuities take on several different appearances. We classify the types of discontinuities we have seen thus far as removable discontinuities, infinite discontinuities, or jump discontinuities. Intuitively, a removable discontinuity is a discontinuity for which there is a hole in the graph, a jump discontinuity is a noninfinite discontinuity for which the sections of the function do not meet up, and an infinite discontinuity is a discontinuity located at a vertical asymptote. [link] illustrates the differences in these types of discontinuities. Although these terms provide a handy way of describing three common types of discontinuities, keep in mind that not all discontinuities fit neatly into these categories.
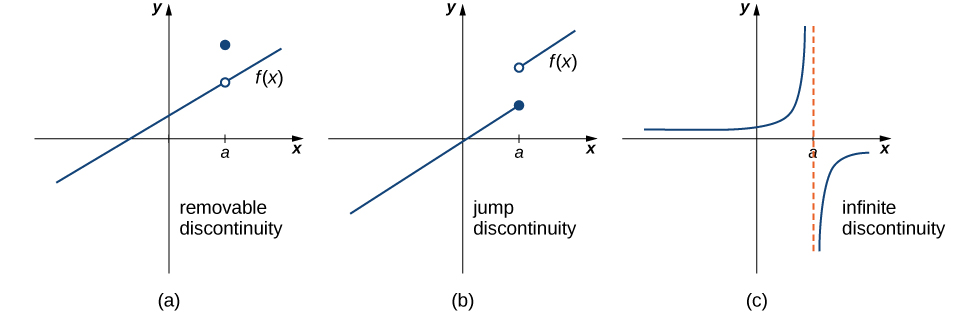
These three discontinuities are formally defined as follows:
If
is discontinuous at a, then
has a removable discontinuity at a if
exists. (Note: When we state that
exists, we mean that
where L is a real number.)
has a jump discontinuity at a if
and
both exist, but
(Note: When we state that
and
both exist, we mean that both are real-valued and that neither take on the values ±∞.)
has an infinite discontinuity at a if
or
In [link], we showed that
is discontinuous at
Classify this discontinuity as removable, jump, or infinite.
To classify the discontinuity at 2 we must evaluate
Since f is discontinuous at 2 and
exists, f has a removable discontinuity at
In [link], we showed that
is discontinuous at
Classify this discontinuity as removable, jump, or infinite.
Earlier, we showed that f is discontinuous at 3 because
does not exist. However, since
and
both exist, we conclude that the function has a jump discontinuity at 3.
Determine whether
is continuous at −1. If the function is discontinuous at −1, classify the discontinuity as removable, jump, or infinite.
The function value
is undefined. Therefore, the function is not continuous at −1. To determine the type of discontinuity, we must determine the limit at −1. We see that
and
Therefore, the function has an infinite discontinuity at −1.
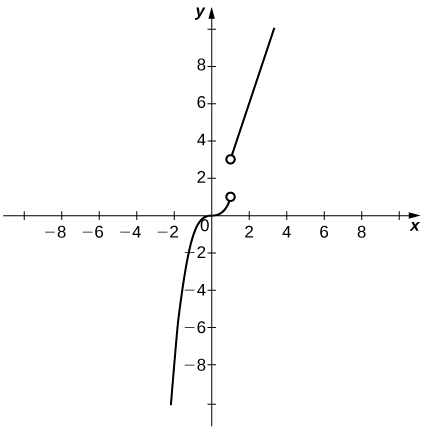
For
decide whether f is continuous at 1. If f is not continuous at 1, classify the discontinuity as removable, jump, or infinite.
Discontinuous at 1; removable
Follow the steps in [link]. If the function is discontinuous at 1, look at
and use the definition to determine the type of discontinuity.
Now that we have explored the concept of continuity at a point, we extend that idea to continuity over an interval. As we develop this idea for different types of intervals, it may be useful to keep in mind the intuitive idea that a function is continuous over an interval if we can use a pencil to trace the function between any two points in the interval without lifting the pencil from the paper. In preparation for defining continuity on an interval, we begin by looking at the definition of what it means for a function to be continuous from the right at a point and continuous from the left at a point.
A function
is said to be continuous from the right at a if
A function
is said to be continuous from the left at a if
A function is continuous over an open interval if it is continuous at every point in the interval. A function
is continuous over a closed interval of the form
if it is continuous at every point in
and is continuous from the right at a and is continuous from the left at b. Analogously, a function
is continuous over an interval of the form
if it is continuous over
and is continuous from the left at b. Continuity over other types of intervals are defined in a similar fashion.
Requiring that
and
ensures that we can trace the graph of the function from the point
to the point
without lifting the pencil. If, for example,
we would need to lift our pencil to jump from
to the graph of the rest of the function over
State the interval(s) over which the function
is continuous.
Since
is a rational function, it is continuous at every point in its domain. The domain of
is the set
Thus,
is continuous over each of the intervals
and
State the interval(s) over which the function
is continuous.
From the limit laws, we know that
for all values of a in
We also know that
exists and
exists. Therefore,
is continuous over the interval
State the interval(s) over which the function
is continuous.
Use [link] as a guide for solving.
The [link] allows us to expand our ability to compute limits. In particular, this theorem ultimately allows us to demonstrate that trigonometric functions are continuous over their domains.
If
is continuous at L and
then
Before we move on to [link], recall that earlier, in the section on limit laws, we showed
Consequently, we know that
is continuous at 0. In [link] we see how to combine this result with the composite function theorem.
Evaluate
The given function is a composite of
and
Since
and
is continuous at 0, we may apply the composite function theorem. Thus,
The proof of the next theorem uses the composite function theorem as well as the continuity of
and
at the point 0 to show that trigonometric functions are continuous over their entire domains.
Trigonometric functions are continuous over their entire domains.
We begin by demonstrating that
is continuous at every real number. To do this, we must show that
for all values of a.
The proof that
is continuous at every real number is analogous. Because the remaining trigonometric functions may be expressed in terms of
and
their continuity follows from the quotient limit law.
□
As you can see, the composite function theorem is invaluable in demonstrating the continuity of trigonometric functions. As we continue our study of calculus, we revisit this theorem many times.
Functions that are continuous over intervals of the form
where a and b are real numbers, exhibit many useful properties. Throughout our study of calculus, we will encounter many powerful theorems concerning such functions. The first of these theorems is the Intermediate Value Theorem.
Let f be continuous over a closed, bounded interval
If z is any real number between
and
then there is a number c in
satisfying
in [link].
![There is a number c∈[a,b] that satisfies f(c)=z. A diagram illustrating the intermediate value theorem. There is a generic continuous curved function shown over the interval [a,b]. The points fa. and fb. are marked, and dotted lines are drawn from a, b, fa., and fb. to the points (a, fa.) and (b, fb.). A third point, c, is plotted between a and b. Since the function is continuous, there is a value for fc. along the curve, and a line is drawn from c to (c, fc.) and from (c, fc.) to fc., which is labeled as z on the y axis.](../resources/CNX_Calc_Figure_02_04_007.jpg)
Show that
has at least one zero.
Since
is continuous over
it is continuous over any closed interval of the form
If you can find an interval
such that
and
have opposite signs, you can use the Intermediate Value Theorem to conclude there must be a real number c in
that satisfies
Note that
and
Using the Intermediate Value Theorem, we can see that there must be a real number c in
that satisfies
Therefore,
has at least one zero.
If
is continuous over
and
can we use the Intermediate Value Theorem to conclude that
has no zeros in the interval
Explain.
No. The Intermediate Value Theorem only allows us to conclude that we can find a value between
and
it doesn’t allow us to conclude that we can’t find other values. To see this more clearly, consider the function
It satisfies
and
For
and
Can we conclude that
has a zero in the interval
No. The function is not continuous over
The Intermediate Value Theorem does not apply here.
Show that
has a zero over the interval
is continuous over
It must have a zero on this interval.
Find
and
Apply the Intermediate Value Theorem.
is continuous at L and
then
For the following exercises, determine the point(s), if any, at which each function is discontinuous. Classify any discontinuity as jump, removable, infinite, or other.
The function is defined for all x in the interval
Removable discontinuity at
infinite discontinuity at
Infinite discontinuity at
Infinite discontinuities at
for
For the following exercises, decide if the function continuous at the given point. If it is discontinuous, what type of discontinuity is it?
at
No. It is a removable discontinuity.
at
at
Yes. It is continuous.
at
at
Yes. It is continuous.
at
In the following exercises, find the value(s) of k that makes each function continuous over the given interval.
In the following exercises, use the Intermediate Value Theorem (IVT).
Let
Over the interval
there is no value of x such that
although
and
Explain why this does not contradict the IVT.
A particle moving along a line has at each time t a position function
which is continuous. Assume
and
Another particle moves such that its position is given by
Explain why there must be a value c for
such that
Since both s and
are continuous everywhere, then
is continuous everywhere and, in particular, it is continuous over the closed interval
Also,
and
Therefore, by the IVT, there is a value
such that
[T] Use the statement “The cosine of t is equal to t cubed.”
Apply the IVT to determine whether
has a solution in one of the intervals
or
Briefly explain your response for each interval.
The function
is continuous over the interval
and has opposite signs at the endpoints.
Consider the graph of the function
shown in the following graph.
![A diagram illustrating the intermediate value theorem. There is a generic continuous curved function shown over the interval [a,b]. The points fa. and fb. are marked, and dotted lines are drawn from a, b, fa., and fb. to the points (a, fa.) and (b, fb.). A third point, c, is plotted between a and b. Since the function is continuous, there is a value for fc. along the curve, and a line is drawn from c to (c, fc.) and from (c, fc.) to fc., which is labeled as z on the y axis.](../resources/CNX_Calc_Figure_02_04_201.jpg)
Let
which makes
continuous for all real numbers? Briefly explain.
a.* * *
b. It is not possible to redefine
since the discontinuity is a jump discontinuity.
Let
for
and
such that
and
and that makes
continuous for all real numbers? Briefly explain.
Sketch the graph of the function
with properties i. through vii.
and
Answers may vary; see the following example:* * *
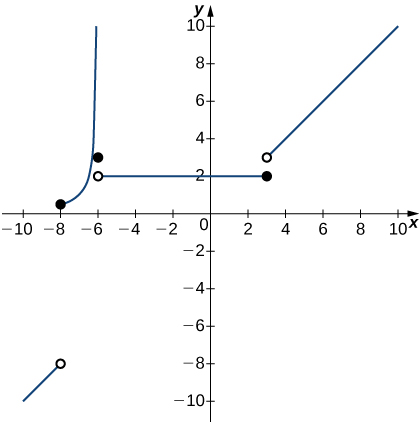
Sketch the graph of the function
with properties i. through iv.
and
exist and are equal.
is left continuous but not continuous at
and right continuous but not continuous at
has a removable discontinuity at
a jump discontinuity at
and the following limits hold:
and
In the following exercises, suppose
is defined for all x. For each description, sketch a graph with the indicated property.
Discontinuous at
with
and
Answers may vary; see the following example:* * *
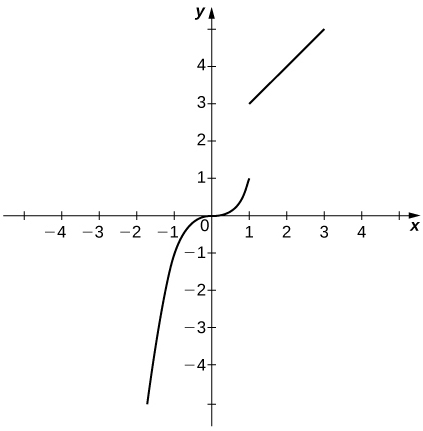
Discontinuous at
but continuous elsewhere with
Determine whether each of the given statements is true. Justify your response with an explanation or counterexample.
is continuous everywhere.
False. It is continuous over
If the left- and right-hand limits of
as
exist and are equal, then f cannot be discontinuous at
If a function is not continuous at a point, then it is not defined at that point.
False. Consider
According to the IVT,
has a solution over the interval
If
is continuous such that
and
have opposite signs, then
has exactly one solution in
False. Consider
on
The function
is continuous over the interval
If
is continuous everywhere and
then there is no root of
in the interval
False. The IVT does not work in reverse! Consider
over the interval
[T] The following problems consider the scalar form of Coulomb’s law, which describes the electrostatic force between two point charges, such as electrons. It is given by the equation
where
is Coulomb’s constant,
are the magnitudes of the charges of the two particles, and r is the distance between the two particles.
To simplify the calculation of a model with many interacting particles, after some threshold value
we approximate F as zero.
and the Coulomb constant
are 1 m apart. Also, assume
How much inaccuracy does our approximation generate? Is our approximation reasonable?
Instead of making the force 0 at R, instead we let the force be 10−20 for
Assume two protons, which have a magnitude of charge
and the Coulomb constant
Is there a value R that can make this system continuous? If so, find it.
Recall the discussion on spacecraft from the chapter opener. The following problems consider a rocket launch from Earth’s surface. The force of gravity on the rocket is given by
where m is the mass of the rocket, d is the distance of the rocket from the center of Earth, and k is a constant.
[T] Determine the value and units of k given that the mass of the rocket on Earth is 3 million kg. (Hint: The distance from the center of Earth to its surface is 6378 km.)
[T] After a certain distance D has passed, the gravitational effect of Earth becomes quite negligible, so we can approximate the force function by
Find the necessary condition D such that the force function remains continuous.
As the rocket travels away from Earth’s surface, there is a distance D where the rocket sheds some of its mass, since it no longer needs the excess fuel storage. We can write this function as
Is there a D value such that this function is continuous, assuming
Prove the following functions are continuous everywhere
For all values of
is defined,
exists, and
Therefore,
is continuous everywhere.
Where is
continuous?
Nowhere
is continuous at a point a if and only if the following three conditions are satisfied: (1)
is defined, (2)
exists, and (3)
is continuous over a closed interval of the form
if it is continuous at every point in
and it is continuous from the right at a and from the left at b
or
if z is any real number between
and
then there is a number c in
satisfying
and
both exist, but
is discontinuous at a, but
exists

You can also download for free at http://cnx.org/contents/9a1df55a-b167-4736-b5ad-15d996704270@5.1
Attribution: