Parametric Equations: Graphs · Algebra and Trigonometry

It is the bottom of the ninth inning, with two outs and two men on base. The home team is losing by two runs. The batter swings and hits the baseball at 140 feet per second and at an angle of approximately

45°

to the horizontal. How far will the ball travel? Will it clear the fence for a game-winning home run? The outcome may depend partly on other factors (for example, the wind), but mathematicians can model the path of a projectile and predict approximately how far it will travel using parametric equations. In this section, we’ll discuss parametric equations and some common applications, such as projectile motion problems.

Graphing Parametric Equations by Plotting Points

In lieu of a graphing calculator or a computer graphing program, plotting points to represent the graph of an equation is the standard method. As long as we are careful in calculating the values, point-plotting is highly dependable.

Sketching the Graph of a Pair of Parametric Equations by Plotting Points

Sketch the graph of the parametric equations $x (t) = t^{2} + 1, y (t) = 2 + t .$

Construct a table of values for $t, x (t),$

and $y (t),$

as in [link], and plot the points in a plane.

t

x (t) = t^{2} + 1

y (t) = 2 + t


$- 5$

26

- 3

- 4

17

- 2

- 3

10

- 1

- 2

5

0

- 1

2

1

0

1

2

1

2

3

2

5

4

3

10

5

4

17

6

5

26

7

The graph is a parabola with vertex at the point $(1, 2),$

opening to the right. See [link].

Graph of the given parabola opening to the right.

Analysis

As values for $t$

progress in a positive direction from 0 to 5, the plotted points trace out the top half of the parabola. As values of $t$

become negative, they trace out the lower half of the parabola. There are no restrictions on the domain. The arrows indicate direction according to increasing values of $t .$

The graph does not represent a function, as it will fail the vertical line test. The graph is drawn in two parts: the positive values for $t,$

and the negative values for $t .$

Sketching the Graph of Trigonometric Parametric Equations

Construct a table of values for the given parametric equations and sketch the graph:

\begin{array}{l} \begin{array}{l} x = 2 \cos t \\ y = 4 \sin t \end{array} \end{array}

Construct a table like that in [link] using angle measure in radians as inputs for $t,$

and evaluating $x$

and $y .$

Using angles with known sine and cosine values for $t$

makes calculations easier.

t

x = 2 \cos t

y = 4 \sin t


0	$x = 2 \cos (0) = 2$

y = 4 \sin (0) = 0

\frac{π}{6}

x = 2 \cos (\frac{π}{6}) = \sqrt{3}

y = 4 \sin (\frac{π}{6}) = 2

\frac{π}{3}

x = 2 \cos (\frac{π}{3}) = 1

y = 4 \sin (\frac{π}{3}) = 2 \sqrt{3}

\frac{π}{2}

x = 2 \cos (\frac{π}{2}) = 0

y = 4 \sin (\frac{π}{2}) = 4

\frac{2 π}{3}

x = 2 \cos (\frac{2 π}{3}) = - 1

y = 4 \sin (\frac{2 π}{3}) = 2 \sqrt{3}

\frac{5 π}{6}

x = 2 \cos (\frac{5 π}{6}) = - \sqrt{3}

y = 4 \sin (\frac{5 π}{6}) = 2

π

x = 2 \cos (π) = - 2

y = 4 \sin (π) = 0

\frac{7 π}{6}

x = 2 \cos (\frac{7 π}{6}) = - \sqrt{3}

y = 4 \sin (\frac{7 π}{6}) = - 2

\frac{4 π}{3}

x = 2 \cos (\frac{4 π}{3}) = - 1

y = 4 \sin (\frac{4 π}{3}) = - 2 \sqrt{3}

\frac{3 π}{2}

x = 2 \cos (\frac{3 π}{2}) = 0

y = 4 \sin (\frac{3 π}{2}) = - 4

\frac{5 π}{3}

x = 2 \cos (\frac{5 π}{3}) = 1

y = 4 \sin (\frac{5 π}{3}) = - 2 \sqrt{3}

\frac{11 π}{6}

x = 2 \cos (\frac{11 π}{6}) = \sqrt{3}

y = 4 \sin (\frac{11 π}{6}) = - 2

2 π

x = 2 \cos (2 π) = 2

y = 4 \sin (2 π) = 0

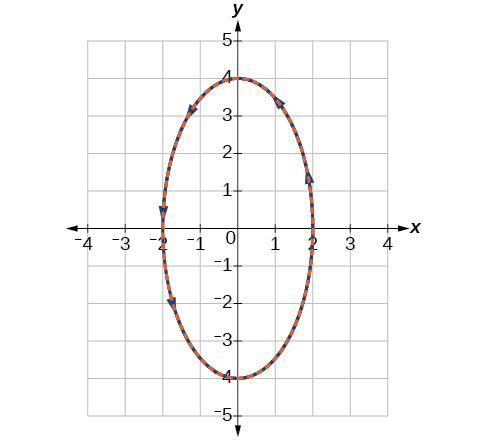
[link] shows the graph.

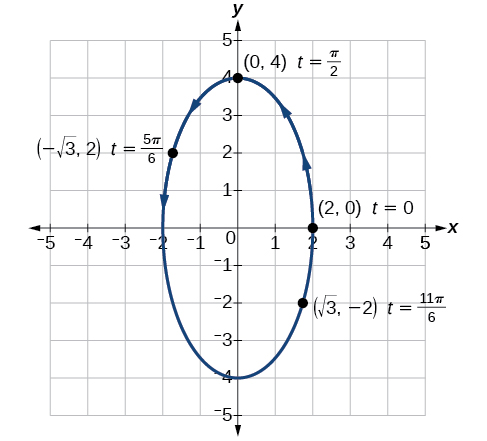
Graph of the given equations - a vertical ellipse.

By the symmetry shown in the values of $x$

and $y,$

we see that the parametric equations represent an ellipse. The ellipse is mapped in a counterclockwise direction as shown by the arrows indicating increasing $t$

values.

Analysis

We have seen that parametric equations can be graphed by plotting points. However, a graphing calculator will save some time and reveal nuances in a graph that may be too tedious to discover using only hand calculations.

Make sure to change the mode on the calculator to parametric (PAR). To confirm, the $Y =$

window should show

\begin{matrix} X_{1 T} = \\ Y_{1 T} = \end{matrix}

instead of $Y_{1} = .$

Graphing Parametric Equations and Rectangular Form Together

Graph the parametric equations $x = 5 \cos t$

and $y = 2 \sin t .$

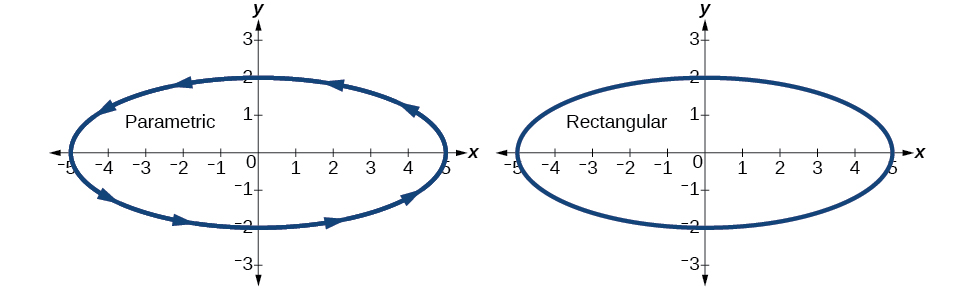
First, construct the graph using data points generated from the parametric form. Then graph the rectangular form of the equation. Compare the two graphs.

Construct a table of values like that in [link].

t

x = 5 \cos t

y = 2 \sin t


$0$

x = 5 \cos (0) = 5

y = 2 \sin (0) = 0

1

x = 5 \cos (1) \approx 2.7

y = 2 \sin (1) \approx 1.7

2

x = 5 \cos (2) \approx −2.1

y = 2 \sin (2) \approx 1.8

3

x = 5 \cos (3) \approx −4.95

y = 2 \sin (3) \approx 0.28

4

x = 5 \cos (4) \approx −3.3

y = 2 \sin (4) \approx −1.5

5

x = 5 \cos (5) \approx 1.4

y = 2 \sin (5) \approx −1.9

−1

x = 5 \cos (−1) \approx 2.7

y = 2 \sin (−1) \approx −1.7

−2

x = 5 \cos (−2) \approx −2.1

y = 2 \sin (−2) \approx −1.8

−3

x = 5 \cos (−3) \approx −4.95

y = 2 \sin (−3) \approx −0.28

−4

x = 5 \cos (−4) \approx −3.3

y = 2 \sin (−4) \approx 1.5

−5

x = 5 \cos (−5) \approx 1.4

y = 2 \sin (−5) \approx 1.9

Plot the $(x, y)$

values from the table. See [link].

Graph of the given ellipse in parametric and rectangular coordinates - it is the same thing in both images.

Next, translate the parametric equations to rectangular form. To do this, we solve for $t$

in either $x (t)$

or $y (t),$

and then substitute the expression for $t$

in the other equation. The result will be a function $y (x)$

if solving for $t$

as a function of $x,$

or $x (y)$

if solving for $t$

as a function of $y .$

\begin{array}{l} x = 5 \cos t \\ \frac{x}{5} = \cos t & Solve for \cos t . \\ y = 2 \sin t \begin{matrix}  \end{matrix} & Solve for \sin t . \\ \frac{y}{2} = \sin t \end{array}

Then, use the Pythagorean Theorem.

\begin{array}{r} \cos^{2} t + \sin^{2} t = 1 \\ {(\frac{x}{5})}^{2} + {(\frac{y}{2})}^{2} = 1 \\ \frac{x^{2}}{25} + \frac{y^{2}}{4} = 1 \end{array}

Analysis

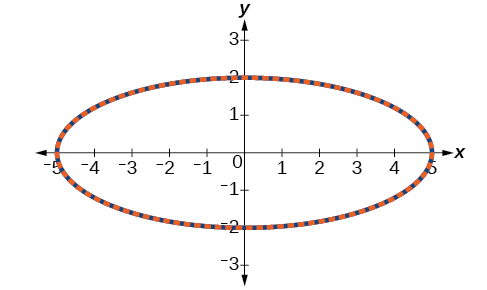
In [link], the data from the parametric equations and the rectangular equation are plotted together. The parametric equations are plotted in blue; the graph for the rectangular equation is drawn on top of the parametric in a dashed style colored red. Clearly, both forms produce the same graph.

Overlayed graph of the two versions of the ellipse, showing that they are the same whether they are given in parametric or rectangular coordinates.

Graphing Parametric Equations and Rectangular Equations on the Coordinate System

Graph the parametric equations $x = t + 1$

and $y = \sqrt{t}, t \geq 0,$

and the rectangular equivalent $y = \sqrt{x - 1}$

on the same coordinate system.

Construct a table of values for the parametric equations, as we did in the previous example, and graph $y = \sqrt{t}, t \geq 0$

on the same grid, as in [link].

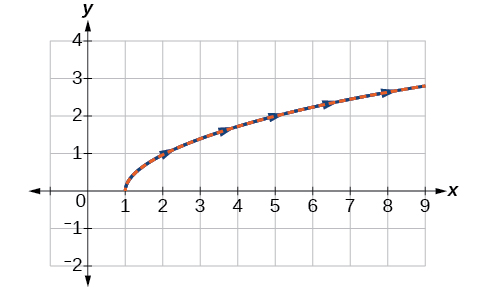
Overlayed graph of the two versions of the given function, showing that they are the same whether they are given in parametric or rectangular coordinates.

Analysis

With the domain on $t$

restricted, we only plot positive values of $t .$

The parametric data is graphed in blue and the graph of the rectangular equation is dashed in red. Once again, we see that the two forms overlap.

Sketch the graph of the parametric equations $x = 2 \cos θ and y = 4 \sin θ,$

along with the rectangular equation on the same grid.

The graph of the parametric equations is in red and the graph of the rectangular equation is drawn in blue dots on top of the parametric equations.

Overlayed graph of the two versions of the ellipse, showing that they are the same whether they are given in parametric or rectangular coordinates.

Applications of Parametric Equations

Many of the advantages of parametric equations become obvious when applied to solving real-world problems. Although rectangular equations in x and y give an overall picture of an object's path, they do not reveal the position of an object at a specific time. Parametric equations, however, illustrate how the values of x and y change depending on t, as the location of a moving object at a particular time.

A common application of parametric equations is solving problems involving projectile motion. In this type of motion, an object is propelled forward in an upward direction forming an angle of

θ

is the initial height of the object. Depending on the units involved in the problem, use

g = 32 ft / s^{2}

Given a projectile motion problem, use parametric equations to solve.

The horizontal distance is given by $x = (v_{0} \cos θ) t .$
Substitute the initial speed of the object for
$v_{0} .$
The expression $\cos θ$
indicates the angle at which the object is propelled. Substitute that angle in degrees for
$\cos θ .$
The vertical distance is given by the formula $y = - \frac{1}{2} g t^{2} + (v_{0} \sin θ) t + h .$
The term
$- \frac{1}{2} g t^{2}$
represents the effect of gravity. Depending on units involved, use
$g = 32 {ft/s}^{2}$
or
$g = 9.8 {m/s}^{2} .$
Again, substitute the initial speed for
$v_{0},$
and the height at which the object was propelled for
$h .$
Proceed by calculating each term to solve for $t .$

Finding the Parametric Equations to Describe the Motion of a Baseball

Solve the problem presented at the beginning of this section. Does the batter hit the game-winning home run? Assume that the ball is hit with an initial velocity of 140 feet per second at an angle of $45°$

to the horizontal, making contact 3 feet above the ground.

Find the parametric equations to model the path of the baseball.
Where is the ball after 2 seconds?
How long is the ball in the air?
Is it a home run?

Use the formulas to set up the equations. The horizontal position is found using the parametric equation for $x .$

Thus,

$\begin{array}{l} x = (v_{0} \cos θ) t \\ x = (140 \cos (45°)) t \end{array}$

The vertical position is found using the parametric equation for $y .$

Thus,

$\begin{array}{l} \begin{array}{l} y = - 16 t^{2} + (v_{0} \sin θ) t + h \\ y = - 16 t^{2} + (140 \sin (45°)) t + 3 \end{array} \end{array}$
Substitute 2 into the equations to find the horizontal and vertical positions of the ball.

$\begin{array}{l} x = (140 \cos (45°)) (2) \\ x = 198 feet \\ y = - 16 {(2)}^{2} + (140 \sin (45°)) (2) + 3 \\ y = 137 feet \end{array}$

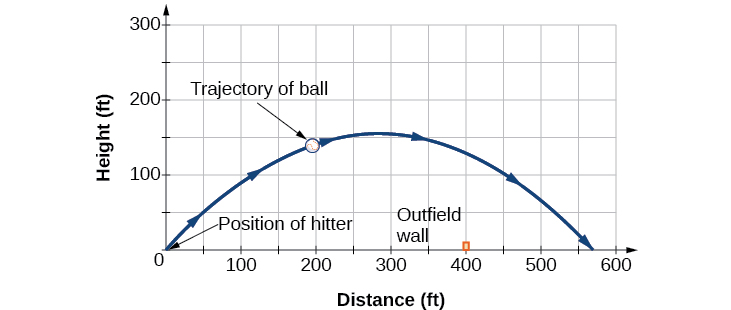
After 2 seconds, the ball is 198 feet away from the batter’s box and 137 feet above the ground.
To calculate how long the ball is in the air, we have to find out when it will hit ground, or when $y = 0.$

Thus,

$\begin{array}{l} y = - 16 t^{2} + (140 \sin (45^{\circ})) t + 3 \\ y = 0 & Set y (t) = 0 and solve the quadratic . \\ t = 6.2173 \end{array}$

When $t = 6.2173$

seconds, the ball has hit the ground. (The quadratic equation can be solved in various ways, but this problem was solved using a computer math program.)
We cannot confirm that the hit was a home run without considering the size of the outfield, which varies from field to field. However, for simplicity’s sake, let’s assume that the outfield wall is 400 feet from home plate in the deepest part of the park. Let’s also assume that the wall is 10 feet high. In order to determine whether the ball clears the wall, we need to calculate how high the ball is when x = 400 feet. So we will set x = 400, solve for $t,$

and input $t$

into $y .$

$\begin{array}{l} \begin{array}{l} x = (140 \cos (45°)) t \\ 400 = (140 \cos (45°)) t \\ t = 4.04 \end{array} \\ \begin{array}{l} y = - 16 {(4.04)}^{2} + (140 \sin (45°)) (4.04) + 3 \\ y = 141.8 \end{array} \end{array}$

The ball is 141.8 feet in the air when it soars out of the ballpark. It was indeed a home run. See [link].

Plotted trajectory of a hit ball, showing the position of the batter at the origin, the ball's path in the shape of a wide downward facing parabola, and the outfield wall as a vertical line segment rising to 10 ft under the ball's path.

Key Concepts

Section Exercises

Verbal

Graphical

For the following exercises, graph each set of parametric equations by making a table of values. Include the orientation on the graph.

For the following exercises, graph the equation and include the orientation. Then, write the Cartesian equation.

For the following exercises, describe the graph of the set of parametric equations.

For the following exercises, use a graphing utility to graph on the window

[- 3, 3]

Technology

For the following exercises, look at the graphs that were created by parametric equations of the form

{\begin{cases} x (t) = a cos (b t) \\ y (t) = c sin (d t) \end{cases} .

Use the parametric mode on the graphing calculator to find the values of

a, b, c,

For the following exercises, use a graphing utility to graph the given parametric equations.

Extensions

For the following exercises, use this scenario: A dart is thrown upward with an initial velocity of 65 ft/s at an angle of elevation of 52°. Consider the position of the dart at any time

t .

For the following exercises, look at the graphs of each of the four parametric equations. Although they look unusual and beautiful, they are so common that they have names, as indicated in each exercise. Use a graphing utility to graph each on the indicated domain.