 <div data-type="example">
<div data-type="example">By the end of this section, you will be able to:
Before you get started, take this readiness quiz.**
We have learned how to solve linear inequalities and rational inequalities previously. Some of the techniques we used to solve them were the same and some were different.
We will now learn to solve inequalities that have a quadratic expression. We will use some of the techniques from solving linear and rational inequalities as well as quadratic equations.
We will solve quadratic inequalities two ways—both graphically and algebraically.
A quadratic equation is in standard form when written as ax2 + bx + c = 0. If we replace the equal sign with an inequality sign, we have a quadratic inequality in standard form.
A quadratic inequality is an inequality that contains a quadratic expression.
The standard form of a quadratic inequality is written:
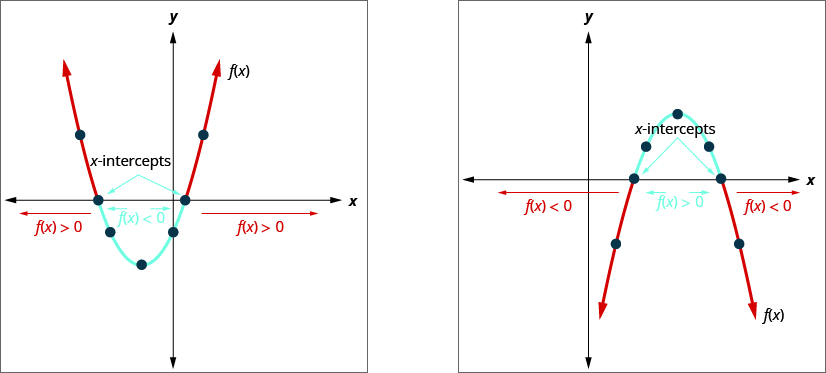
The graph of a quadratic function f(x) = ax2 + bx + c = 0 is a parabola. When we ask when is ax2 + bx + c < 0, we are asking when is f(x) < 0. We want to know when the parabola is below the x-axis.
When we ask when is ax2 + bx + c > 0, we are asking when is f(x) > 0. We want to know when the parabola is above the y-axis.
 <div data-type="example">
<div data-type="example">
Solve
graphically. Write the solution in interval notation.
</div>
ⓐ Solve
graphically and ⓑ write the solution in interval notation.
ⓐ* * *
ⓑ
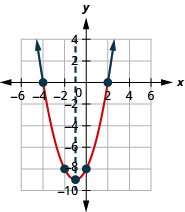
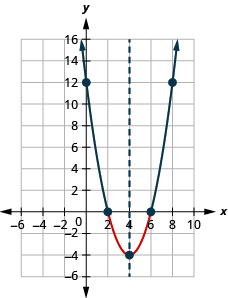
ⓐ Solve
graphically and ⓑ write the solution in interval notation.
ⓐ* * *
ⓑ
We list the steps to take to solve a quadratic inequality graphically.
In the last example, the parabola opened upward and in the next example, it opens downward. In both cases, we are looking for the part of the parabola that is below the x-axis but note how the position of the parabola affects the solution.
Solve
graphically. Write the solution in interval notation.
| The quadratic inequality in standard form. | ||
| Graph the function . | The parabola opens downward. |
|
| Find the line of symmetry. | ||
| Find the vertex. | Vertex |
|
| Find the x-intercepts. Let . | ||
| Factor. Use the Zero Product Property. |
||
| Graph the parabola. | x-intercepts 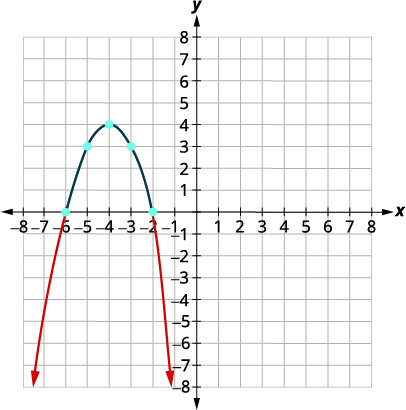
|
|
| Determine the solution from the graph. We include the x-intercepts as the inequality is “less than or equal to.” |
ⓐ Solve
graphically and ⓑ write the solution in interval notation.
ⓐ* * *
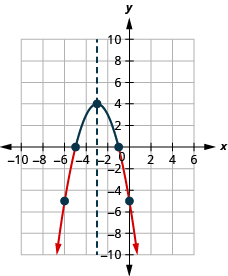
ⓑ
ⓐ Solve
graphically and ⓑ write the solution in interval notation.
ⓐ* * *
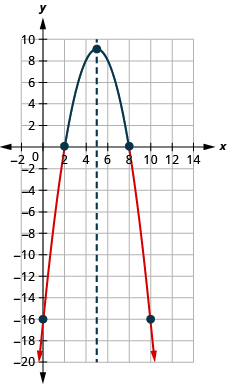
ⓑ
The algebraic method we will use is very similar to the method we used to solve rational inequalities. We will find the critical points for the inequality, which will be the solutions to the related quadratic equation. Remember a polynomial expression can change signs only where the expression is zero.
We will use the critical points to divide the number line into intervals and then determine whether the quadratic expression willl be postive or negative in the interval. We then determine the solution for the inequality.
Solve
algebraically. Write the solution in interval notation.
Solve
algebraically. Write the solution in interval notation.
Solve
algebraically. Write the solution in interval notation.
In this example, since the expression
factors nicely, we can also find the sign in each interval much like we did when we solved rational inequalities. We find the sign of each of the factors, and then the sign of the product. Our number line would like this:
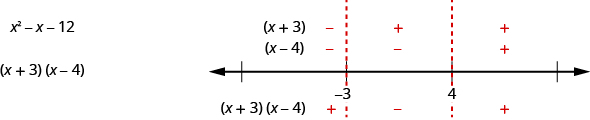 The result is the same as we found using the other method.
The result is the same as we found using the other method.
We summarize the steps here.
Solve
algebraically. Write the solution in interval notation.
| Write the quadratic inequality in standard form. | |
| Multiply both sides of the inequality by . Remember to reverse the inequality sign. |
|
| Determine the critical points by solving the related quadratic equation. |
|
| Write the Quadratic Formula. | |
| Then substitute in the values of . | |
| Simplify. | |
| Simplify the radical. | |
| Remove the common factor, 2. | |
| Use the critical points to divide the number line into intervals. Test numbers from each interval in the original inequality. |

|
| Determine the intervals where the inequality is correct. Write the solution in interval notation. |
in the middle interval |
Solve
algebraically. Write the solution in interval notation.
Solve
algebraically. Write the solution in interval notation.
The solutions of the quadratic inequalities in each of the previous examples, were either an interval or the union of two intervals. This resulted from the fact that, in each case we found two solutions to the corresponding quadratic equation ax2 + bx + c = 0. These two solutions then gave us either the two x-intercepts for the graph or the two critical points to divide the number line into intervals.
This correlates to our previous discussion of the number and type of solutions to a quadratic equation using the discriminant.
For a quadratic equation of the form ax2 + bx + c = 0,
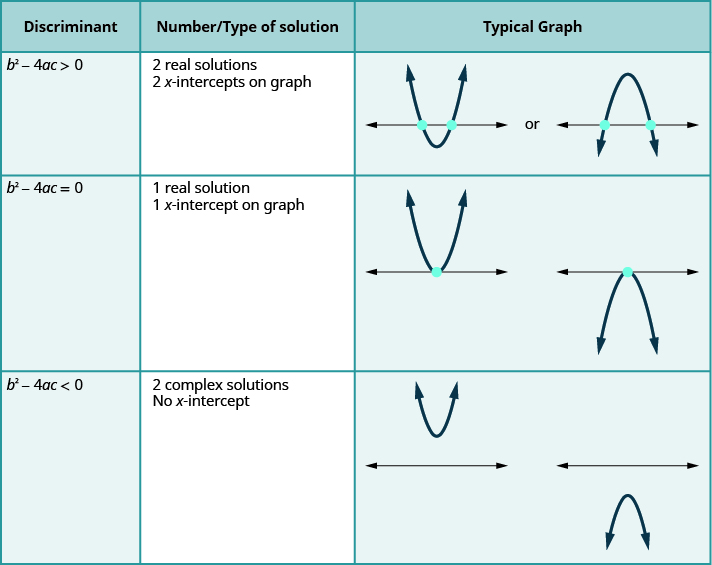 The last row of the table shows us when the parabolas never intersect the x-axis. Using the Quadratic Formula to solve the quadratic equation, the radicand is a negative. We get two complex solutions.
The last row of the table shows us when the parabolas never intersect the x-axis. Using the Quadratic Formula to solve the quadratic equation, the radicand is a negative. We get two complex solutions.
In the next example, the quadratic inequality solutions will result from the solution of the quadratic equation being complex.
Solve, writing any solution in interval notation:
ⓐ
ⓑ
ⓐ* * *
| Write the quadratic inequality in standard form. | |
| Determine the critical points by solving the related quadratic equation. |
|
| Write the Quadratic Formula. | |
| Then substitute in the values of . | |
| Simplify. | |
| Simplify the radicand. | |
| The complex solutions tell us the parabola does not intercept the x-axis. Also, the parabola opens upward. This tells us that the parabola is completely above the x-axis. |
Complex solutions
|
We are to find the solution to
Since for all values of
the graph is above the x-axis, all values of x make the inequality true. In interval notation we write
ⓑ* * *
Since the corresponding quadratic equation is the same as in part (a), the parabola will be the same. The parabola opens upward and is completely above the x-axis—no part of it is below the x-axis.
We are to find the solution to
Since for all values of x the graph is never below the x-axis, no values of x make the inequality true. There is no solution to the inequality.
Solve and write any solution in interval notation:* * *
ⓐ
ⓑ
ⓐ
ⓑ no solution
Solve and write any solution in interval notation:* * *
ⓐ
ⓑ
ⓐ no solution* * *
ⓑ
using properties or transformations.
Solve Quadratic Inequalities Graphically
In the following exercises, ⓐ solve graphically and ⓑ write the solution in interval notation.
ⓐ* * *
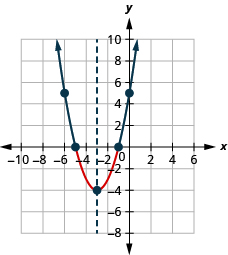
ⓑ
ⓐ* * *
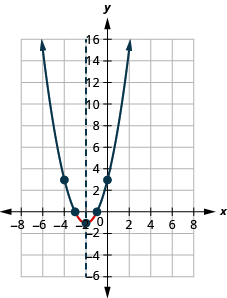
ⓑ
ⓐ* * *
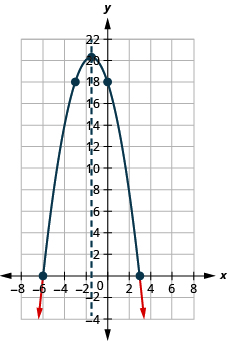
ⓑ
ⓐ* * *
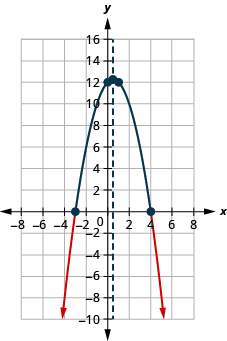
ⓑ
In the following exercises, solve each inequality algebraically and write any solution in interval notation.
no solution
Explain critical points and how they are used to solve quadratic inequalities algebraically.
Answers will vary.
Solve
both graphically and algebraically. Which method do you prefer, and why?
Describe the steps needed to solve a quadratic inequality graphically.
Answers will vary.
Describe the steps needed to solve a quadratic inequality algebraically.
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
 ⓑ On a scale of 1-10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
ⓑ On a scale of 1-10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
Solve Quadratic Equations of the form ax2 = k Using the Square Root Property
In the following exercises, solve using the Square Root Property.
11.
**Solve Quadratic Equations of the Form
Using the Square Root Property**
In the following exercises, solve using the Square Root Property.
Solve Quadratic Equations Using Completing the Square
In the following exercises, complete the square to make a perfect square trinomial. Then write the result as a binomial squared.
In the following exercises, solve by completing the square.
Solve Quadratic Equations of the form ax2 + bx + c = 0 by Completing the Square
In the following exercises, solve by completing the square.
In the following exercises, solve by using the Quadratic Formula.
Use the Discriminant to Predict the Number of Solutions of a Quadratic Equation
In the following exercises, determine the number of solutions for each quadratic equation.
ⓐ
ⓑ
ⓒ
ⓓ
ⓐ 1 ⓑ 2 ⓒ 2 ⓓ 2
ⓐ
ⓑ
ⓒ
ⓓ
Identify the Most Appropriate Method to Use to Solve a Quadratic Equation
In the following exercises, identify the most appropriate method (Factoring, Square Root, or Quadratic Formula) to use to solve each quadratic equation. Do not solve.
ⓐ
ⓑ
ⓒ
ⓐ factor ⓑ Quadratic Formula ⓒ square root
ⓐ
ⓑ
ⓒ
Solve Equations in Quadratic Form
In the following exercises, solve.
Solve Applications Modeled by Quadratic Equations
In the following exercises, solve by using the method of factoring, the square root principle, or the Quadratic Formula. Round your answers to the nearest tenth, if needed.
Find two consecutive odd numbers whose product is 323.
Find two consecutive even numbers whose product is 624.
Two consecutive even numbers whose product is 624 are 24 and 26, and −24 and −26.
A triangular banner has an area of 351 square centimeters. The length of the base is two centimeters longer than four times the height. Find the height and length of the base.
Julius built a triangular display case for his coin collection. The height of the display case is six inches less than twice the width of the base. The area of the of the back of the case is 70 square inches. Find the height and width of the case.
The height is 14 inches and the width is 10 inches.
A tile mosaic in the shape of a right triangle is used as the corner of a rectangular pathway. The hypotenuse of the mosaic is 5 feet. One side of the mosaic is twice as long as the other side. What are the lengths of the sides? Round to the nearest tenth.

A rectangular piece of plywood has a diagonal which measures two feet more than the width. The length of the plywood is twice the width. What is the length of the plywood’s diagonal? Round to the nearest tenth.
The length of the diagonal is 3.6 feet.
The front walk from the street to Pam’s house has an area of 250 square feet. Its length is two less than four times its width. Find the length and width of the sidewalk. Round to the nearest tenth.
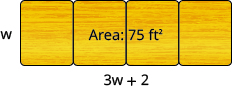
For Sophia’s graduation party, several tables of the same width will be arranged end to end to give serving table with a total area of 75 square feet. The total length of the tables will be two more than three times the width. Find the length and width of the serving table so Sophia can purchase the correct size tablecloth . Round answer to the nearest tenth.
The width of the serving table is 4.7 feet and the length is 16.1 feet.* * *
A ball is thrown vertically in the air with a velocity of 160 ft/sec. Use the formula h = −16t2 + v0t to determine when the ball will be 384 feet from the ground. Round to the nearest tenth.
The couple took a small airplane for a quick flight up to the wine country for a romantic dinner and then returned home. The plane flew a total of 5 hours and each way the trip was 360 miles. If the plane was flying at 150 mph, what was the speed of the wind that affected the plane?
The speed of the wind was 30 mph.
Ezra kayaked up the river and then back in a total time of 6 hours. The trip was 4 miles each way and the current was difficult. If Roy kayaked at a speed of 5 mph, what was the speed of the current?
Two handymen can do a home repair in 2 hours if they work together. One of the men takes 3 hours more than the other man to finish the job by himself. How long does it take for each handyman to do the home repair individually?
One man takes 3 hours and the other man 6 hours to finish the repair alone.
Recognize the Graph of a Quadratic Function
In the following exercises, graph by plotting point.
Graph
Graph
In the following exercises, determine if the following parabolas open up or down.
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ up ⓑ down
Find the Axis of Symmetry and Vertex of a Parabola
In the following exercises, find ⓐ the equation of the axis of symmetry and ⓑ the vertex.
Find the Intercepts of a Parabola
In the following exercises, find the x- and y-intercepts.
Graph Quadratic Functions Using Properties
In the following exercises, graph by using its properties.
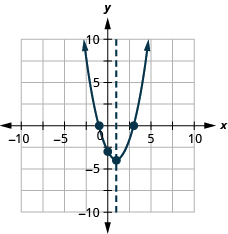
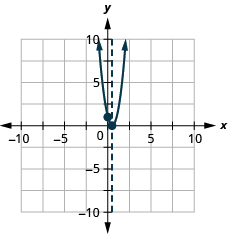

Solve Maximum and Minimum Applications
In the following exercises, find the minimum or maximum value.
The maximum value is 2 when x = 2.
In the following exercises, solve. Rounding answers to the nearest tenth.
A ball is thrown upward from the ground with an initial velocity of 112 ft/sec. Use the quadratic equation h = −16t2 + 112t to find how long it will take the ball to reach maximum height, and then find the maximum height.
A daycare facility is enclosing a rectangular area along the side of their building for the children to play outdoors. They need to maximize the area using 180 feet of fencing on three sides of the yard. The quadratic equation A = −2x2 + 180x gives the area, A, of the yard for the length, x, of the building that will border the yard. Find the length of the building that should border the yard to maximize the area, and then find the maximum area.
The length adjacent to the building is 90 feet giving a maximum area of 4,050 square feet.
**Graph Quadratic Functions of the form
**
In the following exercises, graph each function using a vertical shift.
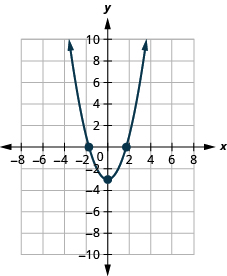
In the following exercises, graph each function using a horizontal shift.
In the following exercises, graph each function using transformations.

**Graph Quadratic Functions of the form
**
In the following exercises, graph each function.
Graph Quadratic Functions Using Transformations
In the following exercises, rewrite each function in the
form by completing the square.
In the following exercises, ⓐ rewrite each function in
form and ⓑ graph it by using transformations.
ⓐ
ⓑ* * *
ⓐ
ⓑ* * *
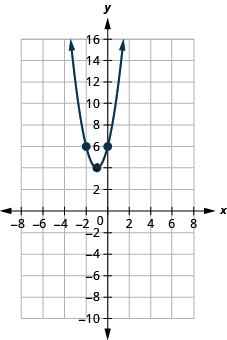
In the following exercises, ⓐ rewrite each function in
form and ⓑ graph it using properties.
ⓐ
ⓑ* * *

Find a Quadratic Function from its Graph
In the following exercises, write the quadratic function in
form.
Solve Quadratic Inequalities Graphically
In the following exercises, solve graphically and write the solution in interval notation.
ⓐ* * *
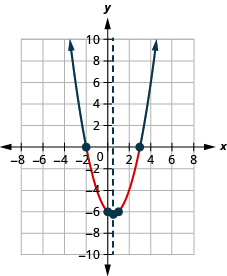
ⓑ
ⓐ* * *
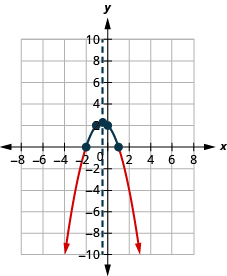
In the following exercises, solve each inequality algebraically and write any solution in interval notation.
no solution
Use the Square Root Property to solve the quadratic equation
Use Completing the Square to solve the quadratic equation
Use the Quadratic Formula to solve the quadratic equation
Solve the following quadratic equations. Use any method.
Use the discriminant to determine the number and type of solutions of each quadratic equation.
2 complex
Solve each equation.
For each parabola, find ⓐ which direction it opens, ⓑ the equation of the axis of symmetry, ⓒ the vertex, ⓓ the x- and y-intercepts, and e) the maximum or minimum value.
ⓐ down ⓑ
ⓒ
ⓓ
ⓔ minimum value of
when
Graph each quadratic function using intercepts, the vertex, and the equation of the axis of symmetry.
In the following exercises, graph each function using transformations.
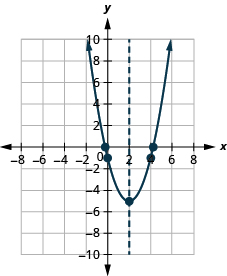
In the following exercises, solve each inequality algebraically and write any solution in interval notation.
Model the situation with a quadratic equation and solve by any method.
Find two consecutive even numbers whose product is 360.
The length of a diagonal of a rectangle is three more than the width. The length of the rectangle is three times the width. Find the length of the diagonal. (Round to the nearest tenth.)
The diagonal is 3.8 units long.
A water balloon is launched upward at the rate of 86 ft/sec. Using the formula h = −16t2 + 86t find how long it will take the balloon to reach the maximum height, and then find the maximum height. Round to the nearest tenth.

You can also download for free at http://cnx.org/contents/02776133-d49d-49cb-bfaa-67c7f61b25a1@4.13
Attribution: