A complex number is of the form a + bi, where a and b are real numbers.
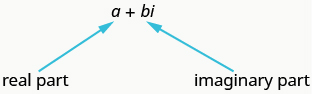
By the end of this section, you will be able to:
Before you get started, take this readiness quiz.
Whenever we have a situation where we have a square root of a negative number we say there is no real number that equals that square root. For example, to simplify
we are looking for a real number x so that x2 = –1. Since all real numbers squared are positive numbers, there is no real number that equals –1 when squared.
Mathematicians have often expanded their numbers systems as needed. They added 0 to the counting numbers to get the whole numbers. When they needed negative balances, they added negative numbers to get the integers. When they needed the idea of parts of a whole they added fractions and got the rational numbers. Adding the irrational numbers allowed numbers like
All of these together gave us the real numbers and so far in your study of mathematics, that has been sufficient.
But now we will expand the real numbers to include the square roots of negative numbers. We start by defining the imaginary unit
as the number whose square is –1.
The imaginary unit i is the number whose square is –1.
We will use the imaginary unit to simplify the square roots of negative numbers.
If b is a positive real number, then
We will use this definition in the next example. Be careful that it is clear that the i is not under the radical. Sometimes you will see this written as
to emphasize the i is not under the radical. But the
is considered standard form.
Write each expression in terms of i and simplify if possible:
ⓐ
ⓑ
ⓒ
ⓐ* * *
ⓑ* * *
ⓒ* * *
Write each expression in terms of i and simplify if possible:
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
Write each expression in terms of i and simplify if possible:
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
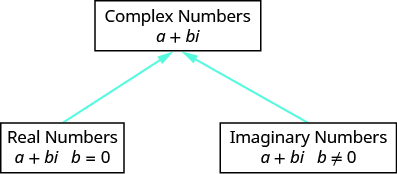
Now that we are familiar with the imaginary number i, we can expand the real numbers to include imaginary numbers. The complex number system includes the real numbers and the imaginary numbers. A complex number is of the form a + bi, where a, b are real numbers. We call a the real part and b the imaginary part.
A complex number is of the form a + bi, where a and b are real numbers.
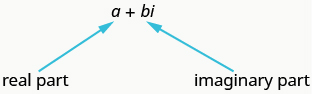
A complex number is in standard form when written as
where a and b are real numbers.
If
then
becomes
and is a real number.
If
then
is an imaginary number.
If
then
becomes
and is called a pure imaginary number.
We summarize this here.
| {: valign=”top”} |
| Real number | |
| {: valign=”top”} |
| Imaginary number | |
| {: valign=”top”} |
| Pure imaginary number | {: valign=”top”}{: .unnumbered summary=”The table has four rows and three columns. The first row is a header and the second column entry a plus b i. In the second row is b equals zero, a plus 0 i, and “Real number”. The third row contains b is not equal to 0, a plus b i, and “Imaginary number”. The fourth row contains a = 0, 0 plus b i, and “Pure imaginary number”.”}
The standard form of a complex number is
so this explains why the preferred form is
when
The diagram helps us visualize the complex number system. It is made up of both the real numbers and the imaginary numbers.
 ### Add or Subtract Complex Numbers
### Add or Subtract Complex Numbers
We are now ready to perform the operations of addition, subtraction, multiplication and division on the complex numbers—just as we did with the real numbers.
Adding and subtracting complex numbers is much like adding or subtracting like terms. We add or subtract the real parts and then add or subtract the imaginary parts. Our final result should be in standard form.
Add:
Add:
Add:
Remember to add both the real parts and the imaginary parts in this next example.
Simplify: ⓐ
ⓑ
ⓐ* * *
ⓑ* * *
Simplify: ⓐ
ⓑ
ⓐ
ⓑ
Simplify: ⓐ
ⓑ
ⓐ
ⓑ
Multiplying complex numbers is also much like multiplying expressions with coefficients and variables. There is only one special case we need to consider. We will look at that after we practice in the next two examples.
Multiply:
Multiply:
Multiply:
In the next example, we multiply the binomials using the Distributive Property or FOIL.
Multiply:
Multiply:
Multiply:
In the next example, we could use FOIL or the Product of Binomial Squares Pattern.
Multiply:
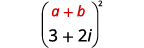 |
|
| Use the Product of Binomial Squares Pattern, |
 |
|
| Simplify. |  |
| Simplify |
 |
|
| Simplify. | 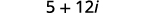 |
Multiply using the Binomial Squares pattern:
Multiply using the Binomial Squares pattern:
Since the square root of a negative number is not a real number, we cannot use the Product Property for Radicals. In order to multiply square roots of negative numbers we should first write them as complex numbers, using
This is one place students tend to make errors, so be careful when you see multiplying with a negative square root.
Multiply:
To multiply square roots of negative numbers, we first write them as complex numbers.
Multiply:
Multiply:
In the next example, each binomial has a square root of a negative number. Before multiplying, each square root of a negative number must be written as a complex number.
Multiply:
To multiply square roots of negative numbers, we first write them as complex numbers.
Multiply:
Multiply:
We first looked at conjugate pairs when we studied polynomials. We said that a pair of binomials that each have the same first term and the same last term, but one is a sum and one is a difference is called a conjugate pair and is of the form
A complex conjugate pair is very similar. For a complex number of the form
its conjugate is
Notice they have the same first term and the same last term, but one is a sum and one is a difference.
A complex conjugate pair is of the form
We will multiply a complex conjugate pair in the next example.
Multiply:
Multiply:
25
Multiply:
29
From our study of polynomials, we know the product of conjugates is always of the form
The result is called a difference of squares. We can multiply a complex conjugate pair using this pattern.
The last example we used FOIL. Now we will use the Product of Conjugates Pattern.
 Notice this is the same result we found in [link].
Notice this is the same result we found in [link].
When we multiply complex conjugates, the product of the last terms will always have an
which simplifies to
This leads us to the Product of Complex Conjugates Pattern:
If a and b are real numbers, then
Multiply using the Product of Complex Conjugates Pattern:
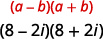
|
|
| Use the Product of Complex Conjugates Pattern, |
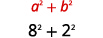
|
| Simplify the squares. |
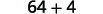
|
| Add. |

|
Multiply using the Product of Complex Conjugates Pattern:
109
Multiply using the Product of Complex Conjugates Pattern:
41
Dividing complex numbers is much like rationalizing a denominator. We want our result to be in standard form with no imaginary numbers in the denominator.
Divide:
Divide:
i
Divide:
i
We summarize the steps here.
Divide, writing the answer in standard form:
Divide, writing the answer in standard form:
Divide, writing the answer in standard form:
Be careful as you find the conjugate of the denominator.
Divide:
Divide:
Divide:
The powers of
make an interesting pattern that will help us simplify higher powers of i. Let’s evaluate the powers of
to see the pattern.
We summarize this now.
If we continued, the pattern would keep repeating in blocks of four. We can use this pattern to help us simplify powers of i. Since i4 = 1, we rewrite each power, in, as a product using i4 to a power and another power of i.
We rewrite it in the form
where the exponent, q, is the quotient of n divided by 4 and the exponent, r, is the remainder from this division. For example, to simplify i57, we divide 57 by 4 and we get 14 with a remainder of 1. In other words,
So we write
and then simplify from there.
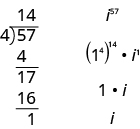 <div data-type="example">
<div data-type="example">
Simplify:
</div>
Simplify:
Simplify:
Access these online resources for additional instruction and practice with the complex number system.
| {: valign=”top”} |
| Real number | |
| {: valign=”top”} |
| Imaginary number | |
| {: valign=”top”} |
| Pure imaginary number | {: valign=”top”}{: summary=”The table has four rows and three columns. The first row is a header and the second column entry a plus b i. In the second row is b equals zero, a plus 0 i, and “Real number”. The third row contains b is not equal to 0, a plus b i, and “Imaginary number”. The fourth row contains a = 0, 0 plus b i, and “Pure imaginary number”.”}

Evaluate the Square Root of a Negative Number
In the following exercises, write each expression in terms of i and simplify if possible.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
Add or Subtract Complex Numbers In the following exercises, add or subtract.
Multiply Complex Numbers
In the following exercises, multiply.
In the following exercises, multiply using the Product of Binomial Squares Pattern.
In the following exercises, multiply.
5
53
In the following exercises, multiply using the Product of Complex Conjugates Pattern.
50
85
Divide Complex Numbers
In the following exercises, divide.
i
Simplify Powers of i
In the following exercises, simplify.
i
1
i
Explain the relationship between real numbers and complex numbers.
Answers will vary.
Aniket multiplied as follows and he got the wrong answer. What is wrong with his reasoning?
Why is
but
Answers will vary.
Explain how dividing complex numbers is similar to rationalizing a denominator.
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
 ⓑ On a scale of
ⓑ On a scale of
how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
Simplify Expressions with Roots
In the following exercises, simplify.
ⓐ
ⓑ
ⓐ 15 ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓒ
ⓐ 2 ⓑ 3 ⓒ 3
ⓐ
ⓑ
ⓒ
Estimate and Approximate Roots
In the following exercises, estimate each root between two consecutive whole numbers.
ⓐ
ⓑ
ⓐ
ⓑ
In the following exercises, approximate each root and round to two decimal places.
ⓐ
ⓑ
ⓒ
Simplify Variable Expressions with Roots
In the following exercises, simplify using absolute values as necessary.
ⓐ
ⓑ
ⓐ a ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓒ
Use the Product Property to Simplify Radical Expressions
In the following exercises, use the Product Property to simplify radical expressions.
ⓐ
ⓑ
ⓐ
ⓑ
In the following exercises, simplify using absolute value signs as needed.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓐ
ⓑ not real
ⓐ
ⓑ
Use the Quotient Property to Simplify Radical Expressions
In the following exercises, use the Quotient Property to simplify square roots.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
**Simplify expressions with
**
In the following exercises, write as a radical expression.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
In the following exercises, write with a rational exponent.
ⓐ
ⓑ
ⓒ
In the following exercises, simplify.
ⓐ
ⓑ
ⓒ
ⓐ 5 ⓑ 3 ⓒ 2
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
**Simplify Expressions with
**
In the following exercises, write with a rational exponent.
ⓐ
ⓑ
ⓒ
In the following exercises, simplify.
ⓐ
ⓑ
ⓒ
ⓐ 125 ⓑ
ⓒ 16
ⓐ
ⓑ
ⓒ
Use the Laws of Exponents to Simplify Expressions with Rational Exponents
In the following exercises, simplify.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
Add and Subtract Radical Expressions
In the following exercises, simplify.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
Multiply Radical Expressions
In the following exercises, simplify.
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
Use Polynomial Multiplication to Multiply Radical Expressions
In the following exercises, multiply.
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
Divide Square Roots
In the following exercises, simplify.
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
Rationalize a One Term Denominator
In the following exercises, rationalize the denominator.
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
ⓐ
ⓑ
ⓒ
Rationalize a Two Term Denominator
In the following exercises, simplify.
Solve Radical Equations
In the following exercises, solve.
no solution
Solve Radical Equations with Two Radicals
In the following exercises, solve.
Use Radicals in Applications
In the following exercises, solve. Round approximations to one decimal place.
Landscaping Reed wants to have a square garden plot in his backyard. He has enough compost to cover an area of 75 square feet. Use the formula
to find the length of each side of his garden. Round your answer to the nearest tenth of a foot.
Accident investigation An accident investigator measured the skid marks of one of the vehicles involved in an accident. The length of the skid marks was 175 feet. Use the formula
to find the speed of the vehicle before the brakes were applied. Round your answer to the nearest tenth.
feet
Evaluate a Radical Function
In the following exercises, evaluate each function.
find* * *
ⓐ
ⓑ
find* * *
ⓐ
ⓑ
ⓐ
ⓑ
find* * *
ⓐ
ⓑ
For the function* * *
find* * *
ⓐ
ⓑ
ⓐ
ⓑ
Find the Domain of a Radical Function
In the following exercises, find the domain of the function and write the domain in interval notation.
Graph Radical Functions
In the following exercises, ⓐ find the domain of the function ⓑ graph the function ⓒ use the graph to determine the range.
ⓐ domain:
ⓑ* * *

ⓒ range:
ⓐ domain:
ⓑ* * *
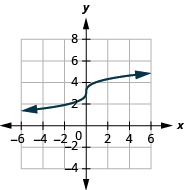
ⓒ range:
Evaluate the Square Root of a Negative Number
In the following exercises, write each expression in terms of i and simplify if possible.
ⓐ
ⓑ
ⓒ
Add or Subtract Complex Numbers
In the following exercises, add or subtract.
Multiply Complex Numbers
In the following exercises, multiply.
In the following exercises, multiply using the Product of Binomial Squares Pattern.
In the following exercises, multiply using the Product of Complex Conjugates Pattern.
Divide Complex Numbers
In the following exercises, divide.
Simplify Powers of i
In the following exercises, simplify.
1
In the following exercises, simplify using absolute values as necessary.
In the following exercises, simplify. Assume all variables are positive.
ⓐ
ⓑ
ⓐ
ⓑ
In the following exercises, solve.
In the following exercise, ⓐ find the domain of the function ⓑ graph the function ⓒ use the graph to determine the range.
ⓐ domain:
ⓑ* * *
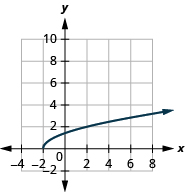
ⓒ range:
is the number whose square is –1. i2 = –1 or
where a, b are real numbers.

You can also download for free at http://cnx.org/contents/02776133-d49d-49cb-bfaa-67c7f61b25a1@4.13
Attribution: