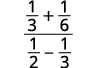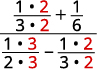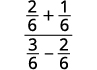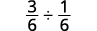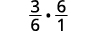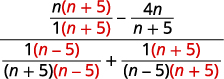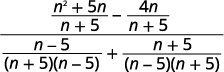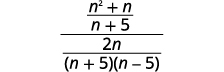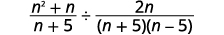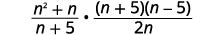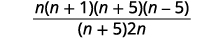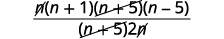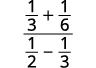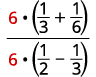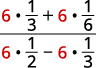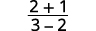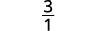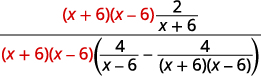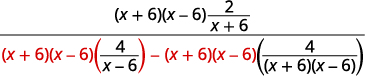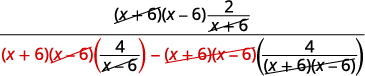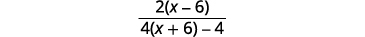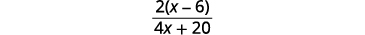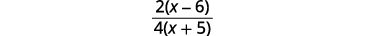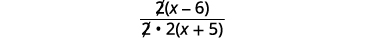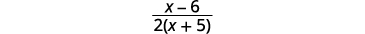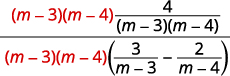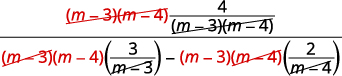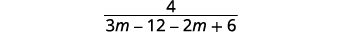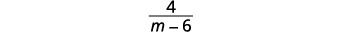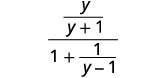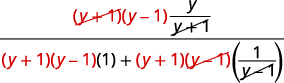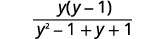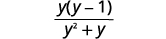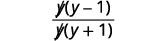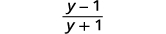Simplify Complex Rational Expressions

Complex fractions are fractions in which the numerator or denominator contains a fraction. We previously simplified complex fractions like these:

In this section, we will simplify complex rational expressions, which are rational expressions with rational expressions in the numerator or denominator.

We noted that fraction bars tell us to divide, so rewrote it as the division problem:

Then, we multiplied the first rational expression by the reciprocal of the second, just like we do when we divide two fractions.

This is one method to simplify complex rational expressions. We make sure the complex rational expression is of the form where one fraction is over one fraction. We then write it as if we were dividing two fractions.

Simplify the complex rational expression by writing it as division: $\frac{\frac{6}{x - 4}}{\frac{3}{x^{2} - 16}} .$

\begin{matrix} \frac{\frac{6}{x - 4}}{\frac{3}{x^{2} - 16}} \\ Rewrite the complex fraction as division. & \frac{6}{x - 4} \div \frac{3}{x^{2} - 16} \\ \begin{matrix} Rewrite as the product of first times the \\ reciprocal of the second. \end{matrix} & \frac{6}{x - 4} \cdot \frac{x^{2} - 16}{3} \\ Factor. & \frac{3 \cdot 2}{x - 4} \cdot \frac{(x - 4) (x + 4)}{3} \\ Multiply. & \frac{3 \cdot 2 (x - 4) (x + 4)}{3 (x - 4)} \\ Remove common factors. & \frac{3 \cdot 2 (x - 4) (x + 4)}{3 (x - 4)} \\ Simplify. & 2 (x + 4) \end{matrix}

Are there any value(s) of x that should not be allowed? The original complex rational expression had denominators of $x - 4$

and $x^{2} - 16 .$

This expression would be undefined if $x = 4$

or $x = −4 .$

Fraction bars act as grouping symbols. So to follow the Order of Operations, we simplify the numerator and denominator as much as possible before we can do the division.

We follow the same procedure when the complex rational expression contains variables.

How to Simplify a Complex Rational Expression using Division

Simplify the complex rational expression by writing it as division: $\frac{\frac{1}{x} + \frac{1}{y}}{\frac{x}{y} - \frac{y}{x}} .$

![Step 1 is to simplify the sum in the numerator and the difference in the denominator of complex rational expression, the quantity 1 divided by x plus 1 divided by y all divided by the quantity x divided by y minus y divided by x. The common denominator of the fractions in the complex rational expression is x y. Multiply the numerator and denominator of 1 divided by x by y over y. Multiply the numerator and denominator of 1 divided by y by x over x. Multiply the numerator and denominator of x divided by y by x over x. Multiply the numerator and denominator of y over x by y over y. The result is the quantity y divided by x y plus x divided by x y all divided by the quantity x squared divided by x y minus y squared divided by x y. Add the fractions in the numerator and subtract the fractions in the denominator. The result is the sum of y and x divided by x y all divided by the difference between x squared and y squared divided by x y. We now have just one rational expression in the numerator and one in the denominator.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_002a_img.jpg) ![Step 2 is to rewrite the complex rational expression as a division problem. Write the numerator divided by the denominator. The result is the quantity of the sum y and x divided by x y all divided by the quantity of the difference between x squared and y squared divided by x y.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_002b_img.jpg) ![Step 3 is to divided the expressions. Multiply the first expression by the reciprocal of the second expression. The result is the quantity of the sum y and x divided by x y times the quantity x y divided by the difference between x squared and y squared. Factor any expressions if possible. The result is the product of x y and the sum of y and x all divided by the product of x y, the difference between x and y, and the sum of x and y. Remove the common factors, x y and the sum of x and y. Simplify. The result is 1 divided by the quantity x minus y.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_002c_img.jpg)

Simplify a Complex Rational Expression by Using the LCD

We “cleared” the fractions by multiplying by the LCD when we solved equations with fractions. We can use that strategy here to simplify complex rational expressions. We will multiply the numerator and denominator by the LCD of all the rational expressions.

Let’s look at the complex rational expression we simplified one way in [link]. We will simplify it here by multiplying the numerator and denominator by the LCD. When we multiply by

\frac{LCD}{LCD}

We will use the same example as in [link]. Decide which method works better for you.

How to Simplify a Complex Rational Expressing using the LCD

Simplify the complex rational expression by using the LCD: $\frac{\frac{1}{x} + \frac{1}{y}}{\frac{x}{y} - \frac{y}{x}} .$

![Step 1 is to find the least common denominator of the complex rational expression, the sum of the quantity 1 divided by x and the quantity 1 divided by y all divided by the difference between the quantity x divided by y and the quantity y divided by x.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_005a_img.jpg) ![Step 2 is to multiply the numerator and the denominator by the least common denominator, x y. The result is x y times the sum of the quantity 1 divided by x and the quantity 1 divided by y all divided by x y times the difference between the quantity x divided by y and the quantity y divided by x.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_005b_img.jpg) ![Step 3 is to simplify the expression. Distribute x y in the numerator and the denominator. The result is x y times 1 divided by x plus x y times 1 divided by y all divided by x y times x divided by y plus x y times y divided by x. It simplifies to the sum of y and x divided by the quantity x squared minus y squared. Write the denominator as the difference of squares, the quantity x minus y times the quantity x plus y. The result is the quantity y plus x all divided by the quantity x minus y times the quantity x plus y. Remove the common factor, y plus x, from the numerator and denominator. The result is 1 divided by the quantity x minus y.](/algebra-intermediate-book/resources/CNX_IntAlg_Figure_07_03_005c_img.jpg)

Be sure to factor the denominators first. Proceed carefully as the math can get messy!

Key Concepts

Practice Makes Perfect

Simplify a Complex Rational Expression by Writing it as Division

In the following exercises, simplify each complex rational expression by writing it as division.

\frac{\frac{2 a}{a + 4}}{\frac{4 a^{2}}{a^{2} - 16}}

\frac{a - 4}{2 a}

\frac{\frac{3 b}{b - 5}}{\frac{b^{2}}{b^{2} - 25}}

\frac{\frac{5}{c^{2} + 5 c - 14}}{\frac{10}{c + 7}}

\frac{1}{2 (c - 2)}

\frac{\frac{8}{d^{2} + 9 d + 18}}{\frac{12}{d + 6}}

\frac{\frac{1}{2} + \frac{5}{6}}{\frac{2}{3} + \frac{7}{9}}

\frac{12}{13}

\frac{\frac{1}{2} + \frac{3}{4}}{\frac{3}{5} + \frac{7}{10}}

\frac{\frac{2}{3} - \frac{1}{9}}{\frac{3}{4} + \frac{5}{6}}

\frac{20}{57}

\frac{\frac{1}{2} - \frac{1}{6}}{\frac{2}{3} + \frac{3}{4}}

\frac{\frac{n}{m} + \frac{1}{n}}{\frac{1}{n} - \frac{n}{m}}

\frac{n^{2} + m}{m - n^{2}}

\frac{\frac{1}{p} + \frac{p}{q}}{\frac{q}{p} - \frac{1}{q}}

\frac{\frac{1}{r} + \frac{1}{t}}{\frac{1}{r^{2}} - \frac{1}{t^{2}}}

\frac{r t}{t - r}

\frac{\frac{2}{v} + \frac{2}{w}}{\frac{1}{v^{2}} - \frac{1}{w^{2}}}

\frac{x - \frac{2 x}{x + 3}}{\frac{1}{x + 3} + \frac{1}{x - 3}}

\frac{(x + 1) (x - 3)}{2}

\frac{y - \frac{2 y}{y - 4}}{\frac{2}{y - 4} + \frac{2}{y + 4}}

\frac{2 - \frac{2}{a + 3}}{\frac{1}{a + 3} + \frac{a}{2}}

\frac{4}{a + 1}

\frac{4 + \frac{4}{b - 5}}{\frac{1}{b - 5} + \frac{b}{4}}

Simplify a Complex Rational Expression by Using the LCD

In the following exercises, simplify each complex rational expression by using the LCD.

\frac{\frac{1}{3} + \frac{1}{8}}{\frac{1}{4} + \frac{1}{12}}

\frac{11}{8}

\frac{\frac{1}{4} + \frac{1}{9}}{\frac{1}{6} + \frac{1}{12}}

\frac{\frac{5}{6} + \frac{2}{9}}{\frac{7}{18} - \frac{1}{3}}

19

\frac{\frac{1}{6} + \frac{4}{15}}{\frac{3}{5} - \frac{1}{2}}

\frac{\frac{c}{d} + \frac{1}{d}}{\frac{1}{d} - \frac{d}{c}}

\frac{c^{2} + c}{c - d^{2}}

\frac{\frac{1}{m} + \frac{m}{n}}{\frac{n}{m} - \frac{1}{n}}

\frac{\frac{1}{p} + \frac{1}{q}}{\frac{1}{p^{2}} - \frac{1}{q^{2}}}

\frac{p q}{q - p}

\frac{\frac{2}{r} + \frac{2}{t}}{\frac{1}{r^{2}} - \frac{1}{t^{2}}}

\frac{\frac{2}{x + 5}}{\frac{3}{x - 5} + \frac{1}{x^{2} - 25}}

\frac{2 x - 10}{3 x + 16}

\frac{\frac{5}{y - 4}}{\frac{3}{y + 4} + \frac{2}{y^{2} - 16}}

\frac{\frac{5}{z^{2} - 64} + \frac{3}{z + 8}}{\frac{1}{z + 8} + \frac{2}{z - 8}}

\frac{3 z - 19}{3 z + 8}

\frac{\frac{3}{s + 6} + \frac{5}{s - 6}}{\frac{1}{s^{2} - 36} + \frac{4}{s + 6}}

\frac{\frac{4}{a^{2} - 2 a - 15}}{\frac{1}{a - 5} + \frac{2}{a + 3}}

\frac{4}{3 a - 7}

\frac{\frac{5}{b^{2} - 6 b - 27}}{\frac{3}{b - 9} + \frac{1}{b + 3}}

\frac{\frac{5}{c + 2} - \frac{3}{c + 7}}{\frac{5 c}{c^{2} + 9 c + 14}}

\frac{2 c + 29}{5 c}

\frac{\frac{6}{d - 4} - \frac{2}{d + 7}}{\frac{2 d}{d^{2} + 3 d - 28}}

\frac{2 + \frac{1}{p - 3}}{\frac{5}{p - 3}}

\frac{2 p - 5}{5}

\frac{\frac{n}{n - 2}}{3 + \frac{5}{n - 2}}

\frac{\frac{m}{m + 5}}{4 + \frac{1}{m - 5}}

\frac{m (m - 5)}{(4 m - 19) (m + 5)}

\frac{7 + \frac{2}{q - 2}}{\frac{1}{q + 2}}

In the following exercises, simplify each complex rational expression using either method.

\frac{\frac{3}{4} - \frac{2}{7}}{\frac{1}{2} + \frac{5}{14}}

\frac{13}{24}

\frac{\frac{v}{w} + \frac{1}{v}}{\frac{1}{v} - \frac{v}{w}}

\frac{\frac{2}{a + 4}}{\frac{1}{a^{2} - 16}}

2 (a - 4)

\frac{\frac{3}{b^{2} - 3 b - 40}}{\frac{5}{b + 5} - \frac{2}{b - 8}}

\frac{\frac{3}{m} + \frac{3}{n}}{\frac{1}{m^{2}} - \frac{1}{n^{2}}}

\frac{3 m n}{n - m}

\frac{\frac{2}{r - 9}}{\frac{1}{r + 9} + \frac{3}{r^{2} - 81}}

\frac{x - \frac{3 x}{x + 2}}{\frac{3}{x + 2} + \frac{3}{x - 2}}

\frac{(x - 1) (x - 2)}{6}

\frac{\frac{y}{y + 3}}{2 + \frac{1}{y - 3}}

Writing Exercises

In this section, you learned to simplify the complex fraction $\frac{\frac{3}{x + 2}}{\frac{x}{x^{2} - 4}}$

two ways: rewriting it as a division problem or multiplying the numerator and denominator by the LCD. Which method do you prefer? Why?

Answers will vary.

Efraim wants to start simplifying the complex fraction $\frac{\frac{1}{a} + \frac{1}{b}}{\frac{1}{a} - \frac{1}{b}}$

by cancelling the variables from the numerator and denominator, $\frac{\frac{1}{a} + \frac{1}{b}}{\frac{1}{a} - \frac{1}{b}} .$

Explain what is wrong with Efraim’s plan.

Self Check

ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

This table has four columns and three rows. The first row is a header and it labels each column, “I can…”, “Confidently,” “With some help,” and “No-I don’t get it!” In row 2, the I can was simplify a complex rational expression by writing it as division. In row 3, the I can was simplify a complex rational expression by using the least common denominator. ⓑ After looking at the checklist, do you think you are well-prepared for the next section? Why or why not?


Simplify the numerator and denominator. Find the LCD and add the fractions in the numerator. Find the LCD and subtract the fractions in the denominator.
Simplify the numerator and denominator.
Rewrite the complex rational expression as a division problem.
Multiply the first by the reciprocal of the second.
Simplify.	3


Simplify the numerator and denominator. Find common denominators for the numerator and denominator.
Simplify the numerators.
Subtract the rational expressions in the numerator and add in the denominator.
Simplify. (We now have one rational expression over one rational expression.)
Rewrite as fraction division.
Multiply the first times the reciprocal of the second.
Factor any expressions if possible.
Remove common factors.
Simplify.


The LCD of all the fractions in the whole expression is 6.
Clear the fractions by multiplying the numerator and denominator by that LCD.
Distribute.
Simplify.


Find the LCD of all fractions in the complex rational expression. The LCD is $x^{2} - 36 = (x + 6) (x - 6)$ .
Multiply the numerator and denominator by the LCD.
Simplify the expression.
Distribute in the denominator.
Simplify.
Simplify.
To simplify the denominator, distribute and combine like terms.
Factor the denominator.
Remove common factors.
Simplify.
Notice that there are no more factors common to the numerator and denominator.


Find the LCD of all fractions in the complex rational expression.
The LCD is $(m - 3) (m - 4) .$
Multiply the numerator and denominator by the LCD.
Simplify.
Simplify.
Distribute.
Combine like terms.


Find the LCD of all fractions in the complex rational expression.
The LCD is $(y + 1) (y - 1) .$
Multiply the numerator and denominator by the LCD.
Distribute in the denominator and simplify.
Simplify.
Simplify the denominator and leave the numerator factored.

Factor the denominator and remove factors common with the numerator.
Simplify.