By the end of this section, you will be able to:
A more thorough introduction to the topics covered in this section can be found in the Elementary Algebra chapter, Foundations.
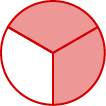
A fraction is a way to represent parts of a whole. The fraction
represents two of three equal parts. See [link]. In the fraction
the 2 is called the numerator and the 3 is called the denominator. The line is called the fraction bar.

A fraction is written
where
and
a is the numerator and b is the denominator.
A fraction represents parts of a whole. The denominator
is the number of equal parts the whole has been divided into, and the numerator
indicates how many parts are included.
Fractions that have the same value are equivalent fractions. The Equivalent Fractions
Property allows us to find equivalent fractions and also simplify fractions.
If a, b, and c are numbers where
then
and
A fraction is considered simplified if there are no common factors, other than 1, in its numerator and denominator.
For example,
is simplified because there are no common factors of 2 and
is not simplified because 5 is a common factor of 10 and
We simplify, or reduce, a fraction by removing the common factors of the numerator and denominator. A fraction is not simplified until all common factors have been removed. If an expression has fractions, it is not completely simplified until the fractions are simplified.
Sometimes it may not be easy to find common factors of the numerator and denominator. When this happens, a good idea is to factor the numerator and the denominator into prime numbers. Then divide out the common factors using the Equivalent Fractions Property.
Simplify:
Simplify:
Simplify:
We now summarize the steps you should follow to simplify fractions.
If needed, factor the numerator and denominator into prime numbers first.
Many people find multiplying and dividing fractions easier than adding and subtracting fractions.
To multiply fractions, we multiply the numerators and multiply the denominators.
If a, b, c, and d are numbers where
and
then
To multiply fractions, multiply the numerators and multiply the denominators.
When multiplying fractions, the properties of positive and negative numbers still apply, of course. It is a good idea to determine the sign of the product as the first step. In [link], we will multiply negative and a positive, so the product will be negative.
When multiplying a fraction by an integer, it may be helpful to write the integer as a fraction. Any integer, a, can be written as
So, for example,
Multiply:
The first step is to find the sign of the product. Since the signs are the same, the product is positive.
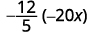 |
|
| Determine the sign of the product. The signs are the same, so the product is positive. |
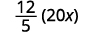 |
| Write 20x as a fraction. | 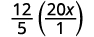 |
| Multiply. | 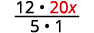 |
| Rewrite 20 to show the common factor 5 and divide it out. |
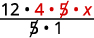 |
| Simplify. | 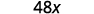 |
Multiply:
Multiply:
Now that we know how to multiply fractions, we are almost ready to divide. Before we can do that, we need some vocabulary. The reciprocal of a fraction is found by inverting the fraction, placing the numerator in the denominator and the denominator in the numerator. The reciprocal of
is
Since 4 is written in fraction form as
the reciprocal of 4 is
To divide fractions, we multiply the first fraction by the reciprocal of the second.
If a, b, c, and d are numbers where
and
then
To divide fractions, we multiply the first fraction by the reciprocal of the second.
We need to say
and
to be sure we don’t divide by zero!
Find the quotient:
 |
|
| To divide, multiply the first fraction by the reciprocal of the second. |
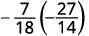 |
| Determine the sign of the product, and then multiply. |
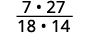 |
| Rewrite showing common factors. | 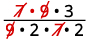 |
| Remove common factors. | 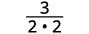 |
| Simplify. | 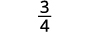 |
Divide:
Divide:
The numerators or denominators of some fractions contain fractions themselves. A fraction in which the numerator or the denominator is a fraction is called a complex fraction.
A complex fraction is a fraction in which the numerator or the denominator contains a fraction.
Some examples of complex fractions are:
To simplify a complex fraction, remember that the fraction bar means division. For example, the complex fraction
means
Simplify:
Simplify:
Simplify:
When we multiplied fractions, we just multiplied the numerators and multiplied the denominators right straight across. To add or subtract fractions, they must have a common denominator.
If a, b, and c are numbers where
then
To add or subtract fractions, add or subtract the numerators and place the result over the common denominator.
The least common denominator (LCD) of two fractions is the smallest number that can be used as a common denominator of the fractions. The LCD of the two fractions is the least common multiple (LCM) of their denominators.
The least common denominator (LCD) of two fractions is the least common multiple (LCM) of their denominators.
After we find the least common denominator of two fractions, we convert the fractions to equivalent fractions with the LCD. Putting these steps together allows us to add and subtract fractions because their denominators will be the same!
Add:
Add:
Add:
We now have all four operations for fractions. [link] summarizes fraction operations.
| Fraction Multiplication | Fraction Division |
| Multiply the numerators and multiply the denominators | Multiply the first fraction by the reciprocal of the second. |
| Fraction Addition | Fraction Subtraction |
| Add the numerators and place the sum over the common denominator. | Subtract the numerators and place the difference over the common denominator. |
| To multiply or divide fractions, an LCD is NOT needed. To add or subtract fractions, an LCD is needed. |
|
When starting an exercise, always identify the operation and then recall the methods needed for that operation.
Simplify: ⓐ
ⓑ
First ask, “What is the operation?” Identifying the operation will determine whether or not we need a common denominator. Remember, we need a common denominator to add or subtract, but not to multiply or divide.
ⓐ* * *
ⓑ* * *
Notice, we needed an LCD to add
but not to multiply
Simplify: ⓐ
ⓑ
ⓐ
ⓑ
Simplify: ⓐ
ⓑ
ⓐ
ⓑ
The fraction bar in a fraction acts as grouping symbol. The order of operations then tells us to simplify the numerator and then the denominator. Then we divide.
Where does the negative sign go in a fraction? Usually the negative sign is in front of the fraction, but you will sometimes see a fraction with a negative numerator, or sometimes with a negative denominator. Remember that fractions represent division. When the numerator and denominator have different signs, the quotient is negative.
For any positive numbers a and b,
Simplify:
The fraction bar acts like a grouping symbol. So completely simplify the numerator and the denominator separately.
Simplify:
4
Simplify:
2
Now we’ll look at complex fractions where the numerator or denominator contains an expression that can be simplified. So we first must completely simplify the numerator and denominator separately using the order of operations. Then we divide the numerator by the denominator as the fraction bar means division.
Simplify:
Simplify:
Simplify:
272
Simplify:
It may help to put parentheses around the numerator and the denominator.
Simplify:
2
Simplify:
We have evaluated expressions before, but now we can evaluate expressions with fractions. Remember, to evaluate an expression, we substitute the value of the variable into the expression and then simplify.
Evaluate
when
and
Substitute the values into the expression.
 |
|
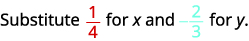 |
 |
| Simplify exponents first. | 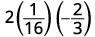 |
| Multiply; divide out the common factors. Notice we write 16 as to make it easy to remove common factors. |
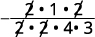 |
| Simplify. | 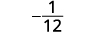 |
Evaluate
when
and
Evaluate
when
and
Access this online resource for additional instruction and practice with fractions.
If a, b, and c are numbers where
then
If needed, factor the numerator and denominator into prime numbers first.
If a, b, c, and d are numbers where
and
then
To multiply fractions, multiply the numerators and multiply the denominators.
If a, b, c, and d are numbers where
and
then
To divide fractions, we multiply the first fraction by the reciprocal of the second.
If a, b, and c are numbers where
then
To add or subtract fractions, add or subtract the numerators and place the result over the common denominator.
For any positive numbers a and b,
Simplify Fractions
In the following exercises, simplify.
Multiply and Divide Fractions
In the following exercises, perform the indicated operation.
In the following exercises, simplify.
Add and Subtract Fractions
In the following exercises, add or subtract.
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
ⓐ
ⓑ
Use the Order of Operations to Simplify Fractions
In the following exercises, simplify.
Mixed Practice
In the following exercises, simplify.
Evaluate Variable Expressions with Fractions
In the following exercises, evaluate.
when* * *
ⓐ
ⓑ
ⓐ
ⓑ
when* * *
ⓐ
ⓑ
when* * *
and
when* * *
and
when* * *
when* * *
Why do you need a common denominator to add or subtract fractions? Explain.
Answers will vary.
How do you find the LCD of 2 fractions?
Explain how you find the reciprocal of a fraction.
Answers will vary.
Explain how you find the reciprocal of a negative number.
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
 ⓑ What does this checklist tell you about your mastery of this section? What steps will you take to improve?
ⓑ What does this checklist tell you about your mastery of this section? What steps will you take to improve?
where
the denominator b is the number of equal parts the whole has been divided into.
where
and a is the numerator and b is the denominator. A fraction represents parts of a whole.
where
the numerator a indicates how many parts are included.

You can also download for free at http://cnx.org/contents/02776133-d49d-49cb-bfaa-67c7f61b25a1@4.13
Attribution: